Final

Ver más

Macromolecues

por Sage LI

Navigation Systems

por Berta Alomà Sesé

Introduction to functions.

por Mya Morgan - Sandalwood Heights SS (2442)

php & MySQL

por Piotr Delikat

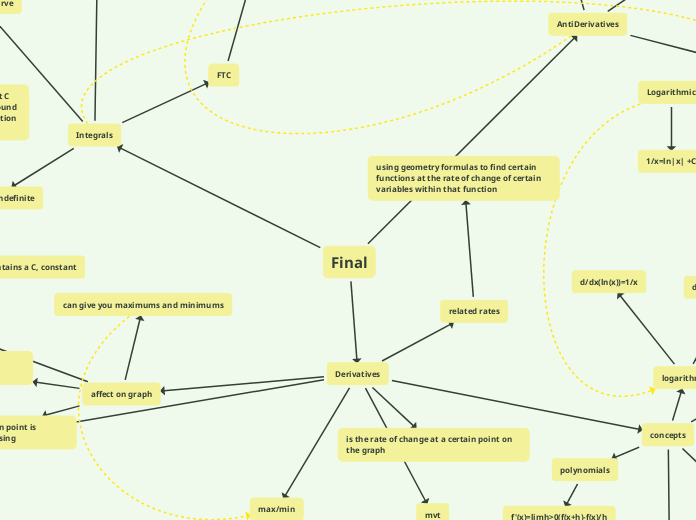
Final

Derivatives

mvt

as long as a function is differentiable and continuous than the derivative can be used to see that c exists on (a,b)

max/min

minimums can be determined where the derivative at a certain point =0, the point before being negative and the point after being positive

maximums can be determined where the derivative at a certain point =0, the point before being positive and the point after being negative

related rates

using geometry formulas to find certain functions at the rate of change of certain variables within that function

implicit differentation

can be used to find the slope at a certain point in a graph

used to find the derivative of equations not written in terms of one variable

affect on graph

concavity

found using the derivative of the derivative

if at a point on the 2nd derivative the value is positive the function is concave up

if at a point on the 2nd derivative the value is negative the function is concave down

if the derivative at a certain point is positive the slope is increasing

if the derivative at a certain point is negative than the slope is decreasing

can give you maximums and minimums

concepts

trig

logarithmic

d/dx(loga(x))=1/xln(a)

d/dx(ln(x))=1/x

chain rule

F'(x)=f'(g(x))g'(x)

inverse trig

http://www.beyondcalculus.com/derivatives/functions.html

Product rule

d/dx(f(x)*g(x)= f'(x)*g(x)+f(x)*g'(x)

Quotient rule

d/dx(f(x)/g(x))= (f'*g-f*g')/g(x)^2

exponential

d/dx(b^x)=b^x*ln(b)

polynomials

f'(x)=limh>0(f(x+h)-f(x)/h

is the rate of change at a certain point on the graph

Integrals

Net Change Theorem

area under curve

can be used to find net area under a cover from (a,b)

finding antiderivate of inside function of integral then finding F(a)-F(b)= the net area

definite vs indefinite

Definite solution does not contain that C rather an exact constant and can be found by knowing an exact point on the function and filling it in.

indefinite solution contains a C, constant

FTC

if the function is defined between (a,b) then the integral if the function is the antiderivative

AntiDerivatives

+C, the constant

is apart of the general solution

rules

kdx=kx+C

x^n=1/n+1x^n+1+C

e^ax=1/a*e^ax+C

b^x=1/lnb*b^x+C

Logarithmic

1/x=ln|x| +C

exact opposite of derivative

could just be opposite of the derivative but dont forget the +C