Scientific Notation
Definition
Scientific notation is a way of writing very large numbers in a more convenient way. We usally right in the expanded/standard form, however scientific notation uses a multiplication by ten (x10) with exponents to reduce the characters in a number.
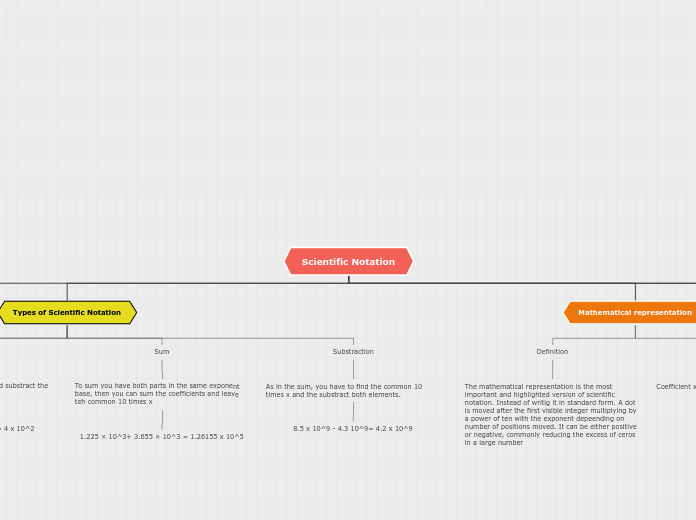
Types of Scientific Notation
Multiplication
To multiply innscientific notation, multiply the coefficients and sum the exponents.
(4.3 × 10^8) (5.6 × 10^4) = 24.08 x 10^12
Division
To divide, divide the coefficients and substract the exponents
10.4 × 10^6/ 5.2 × 10^4 = 4 x 10^2
Sum
To sum you have both parts in the same exponent base, then you can sum the coefficients and leave teh common 10 times x
1.225 × 10^3+ 3.655 × 10^3 = 1.26155 x 10^5
Substraction
As in the sum, you have to find the common 10 times x and the substract both elements.
8.5 x 10^9 - 4.3 10^9= 4.2 x 10^9
Mathematical representation
Definition
The mathematical representation is the most important and highlighted version of scientific notation. Instead of writig it in standard form. A dot is moved after the first visible integer multiplying by a power of ten with the exponent depeending on number of positions moved. It can be either positive or negative, commonly reducing the excess of ceros in a large number
Examples
Coefficient x 10^times moved as in N x 10^e
Graphical representation
Definition
Since the scientific notation is used to represent numbers, their graphical representation is not very different. They can be used as coordinates to representa point, or even be included in a function although this might be very big or very large. They however are not represented ver often in a graph
Examples
6.5 ✕ 10^8
700 = 7 × 10^2
3.1416 × 10^0