MAT.126
2.1-2.2
2.2 Basic Differentiation Rules and Rates of Change
Use derivatives to find rates of change
Average velocity = secant line (no limit)
Instantaneous velocity = tangent line (limit)
Speed is the absolute value of velocity (velocity is a vector quantity).
Find the derivatives of the sine function and of the cosine function
d/dx[sin x] = cos x
d/dx[cos x] = -sin x
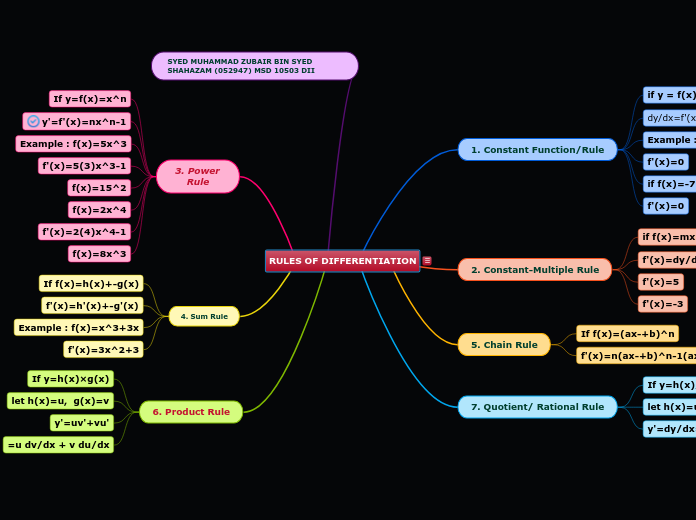
Find the derivative of a function using the Sum and Difference Rules
The sum (or difference) of two differentiable functions f and g is itself differentiable. Moreover, the derivative of f+g (or f-g) is the sum (or difference) of the derivatives of f and g.
Find the derivative of a function using the Constant Multiple Rule
If f is a differentiable function and c is a real number, then cf is also differentiable and d/dx[cf(x)]=cf'(x).
Using parentheses when differentiating
Find the derivative of a function using the Power Rule
The derivative of a power function x^n is nx^{n-1} for n a rational number.
For f to be differentiable at x=0, n must be a number such that x^{n-1} is defined on an interval containing 0.
Finding the equation of a tangent line
Evaluating the derivative to find the slope at a point
Rewriting
It is very useful to rewrite radicals into rational exponent form and to write variables in the denominator of a fraction in negative exponent form.
x
The derivative of x is 1.
This follows from the fact that the slope of the line y=x is 1.
Find the derivative of a function using the Constant Rule
The derivative of a constant function is 0.
This means that the slope of a constant function is 0.
2.1 The Derivative and the Tangent Line Problem
The derivative is important to three of the four major problems that led to the development of calculus.
The tangent line problemThe velocity and acceleration problemThe minimum and maximum problemUnderstand the relationship between differentiability and continuity
Theorem 2.1
Differentiability Imples Continuity
If f is differentiable at x=c, then f is continuous at x=c.
The converse is not true: continuity does not imply differentiability.
Differentiability on a closed interval
f is differentiable on the closed interval [a,b] if it is differentiable on the open interval (a,b) and if the derivative from the right at a and the derivative from the left at b both exist.
Derivatives from the left and from the right
f is differentiable at c if the left derivative at c and the right derivative at c both exist and are equal to the same value.
Alternate form
f(x) - f(c)
f'(c) = lim -----------
x to c x - c
Use the limit definition to find the derivative of a function
The derivative of f at x is given by f'(x) = the limit as h goes to zero of the slope of the secant line for any fixed point (x,f(x)) provided the limit exists.
For all x for which this limit exists, f' is a function of x.
Notation
dy d
f'(x) = -- = y' = ---[f(x)] = D_x[y]
dx dx
f prime of x
derivative of y with respect to x; dy, dx
y prime
derivative of f(x) with respect to x
Vocabulary
The process of finding the derivative of a function is called differentiation.
A function is differentiable at x if its derivative exists at x.
A function is differentiable on an open interval (a,b) if it is differentiable at every point in the interval.
Find the slope of the tangent line to a curve at a point
The tangent line is difficult to define for general curves.
Tangent Lines
We will define the tangent line to the curve f at the point (c,f(c)) as the line with slope equal to the limit as h goes to zero of the secant slope with fixed point (c,f(c)) (assuming the limit exists) that passes through the point (c,f(c)).
The slope of the tangent line to the graph of f at the point (c,f(c)) is also called the slope of the graph of f at x=c.
If the limit as h goes to zero of the secant slope is positive infinity or negative infinity, then the tangent line is a vertical line and its equation is x=c.
Slope of a secant line
We can define the slope of a secant line as
f(c+h) - f(c)
m_{sec} = -------------
h
Secant lines
Secant lines are lines that intersect a curve at two points.
Secant lines with the two points very close together can be used to approximate a tangent line.
But no matter how close the two points are, the secant line is still only an approximation to the desired tangent line.