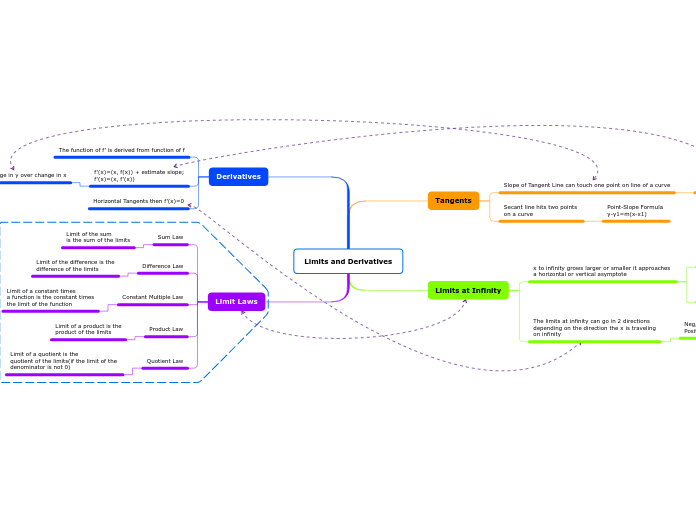
Limits and Derivatives

Slope of Tangent Line can touch one point on line of a curve

2 points to estimate a slope

Secant line hits two points
on a curve

Point-Slope Formula
y-y1=m(x-x1)

x to infinity grows larger or smaller it approaches
a horizontal or vertical asymptote

As x approaches a number and f(x) is
equal to infinity than the function
has a vertical asymptote

As x approaches infinity and f(x)
equals a number than the function has a
horizontal asymptote

The limits at infinity can go in 2 directions
depending on the direction the x is traveling
on infinity

Negative means x is coming from the left
Positive means x is coming from the right

The function of f' is derived from function of f

f'(x)=(x, f(x)) + estimate slope;
f'(x)=(x, f'(x))

Slope change in y over change in x

Horizontal Tangents then f'(x)=0

Sum Law

Limit of the sum
is the sum of the limits

Difference Law

Limit of the difference is the
difference of the limits

Constant Multiple Law

Limit of a constant times
a function is the constant times
the limit of the function

Product Law

Limit of a product is the
product of the limits

Quotient Law

Limit of a quotient is the
quotient of the limits(if the limit of the
denominator is not 0)