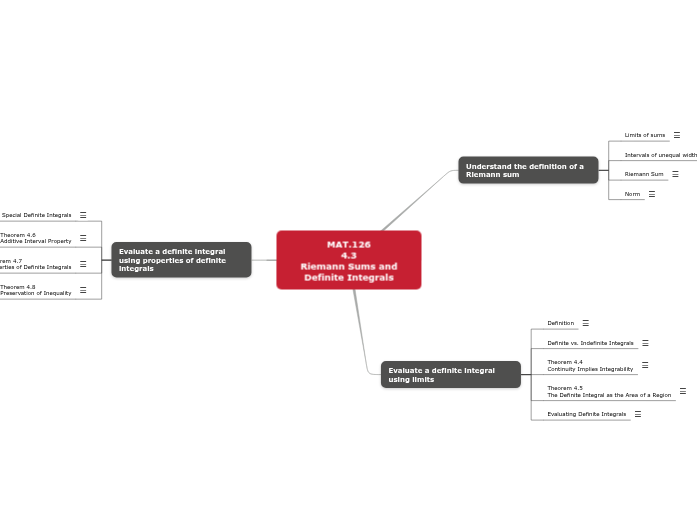
MAT.126
4.3
Riemann Sums and Definite Integrals
Understand the definition of a Riemann sum
Limits of sums
Intervals of unequal width
Riemann Sum
Norm
Evaluate a definite integral using properties of definite integrals
Definitions of Two Special Definite Integrals
Theorem 4.6
Additive Interval Property
Theorem 4.7
Properties of Definite Integrals
Theorem 4.8
Preservation of Inequality
Evaluate a definite integral using limits
Definition
Definite vs. Indefinite Integrals
Theorem 4.4
Continuity Implies Integrability
Theorem 4.5
The Definite Integral as the Area of a Region
Evaluating Definite Integrals