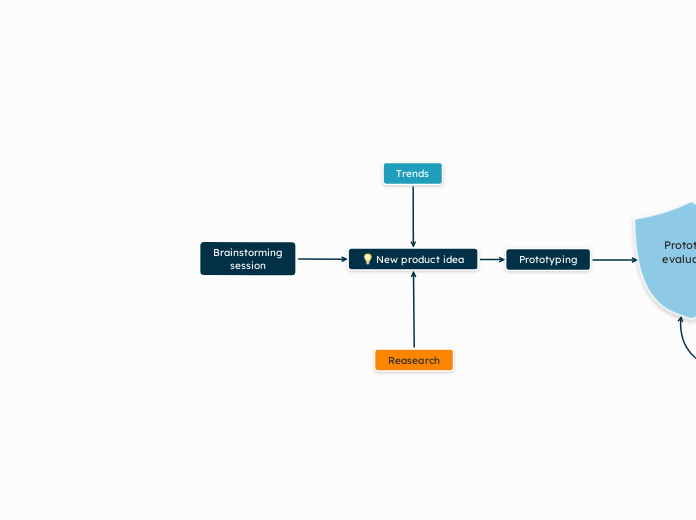
Characteristics of a Successful Coach:
1. Deep content knowledge
2. Skill in various pedagogies
3. Human Relational Skills
4. Knowledge of research and data analysis practices.
5. Flexibility
(Huetinck and Munshin, 2007, p. 433)
Evaluators
Students
Class Discussion
Peers
Self
Class discussion
Weekly Log
Review of videotape.
Student Feedback
Reflection on Daily Lesson Plans
Supervisors
"All persons concerned with education - including students, parents, teachers, administrators, policy-makers, university faculty, families and community members - must work together to make this vision of mathematics teaching and learning a reality (Huetinck and Munshin, 2008, p. 419)
Parts 2 and 3 are divided into four grade bands: preK-2, 3-5, 6-8, and 9-12.
Differentiated Learning
Performance assessment in mathematics = "criterion referenced, that is, an individual's performance is compared to a specific learning objective or performance is compared to a specific learning objective or performance standard;" (Huetinck & Mushin, 2008, p. 358) Observing and interviewing students involvement, understanding, and ending product of the mathematical task, project, or investigation.
Developing performance task
Step 5: Revise
Student work that falls below the minimum standard is returned, with specific suggestions concerning the nature of the improvement needed to being the work up to standard
Step 4: Score Student Work
Delineates the key components of each rubric level with sufficient specificity to explain a given score
Step 3: Identify Anchor Papers
Provide "anchor papers" (examples) that exemplifies the different performance levels, as established by the rubric
For situations, such as an ongoing project, providing check-points to mark student progress toward the end goal
Step 2: Developing Rubrics
Develop holistic rubrics to use when scoring. It is a good idea to create the rubric before giving the task to the students, because the instructor can prepare for any misconceptions students may have
identify specific differences between an unacceptable response and one that is filled with errors/misunderstandings
identify key mathematical elements that determine what is acceptable
Step 1: Identify Outcomes
The task stems from the curriculum and demonstrate understanding of mathematical concepts, using multiple problem-solving strategies, and connecting mathematics to other topics/subjects
Clear learning goals for the class and for the given activity, so students know what is expected
Performance Tasks
Specify and identify the characteristics of the different levels of the rubric, keeping in mind the mathematical objectives you are teaching
Assessments measures student performance. Hence, students are expected to show reasoning, justify approaches, and arrive at a product that meets given criteria (Huetinck & Mushin, 2008, p. 357)
Does not replace standardized test. Provide direct information to the teacher, students, and families about the mathematics a student is able to understand and use
Student involvement in the development of rubrics is important to the instructional design in the class
help both teachers and students gain useful information to improve achievement
students compared to a minimum level of proficiency instead of one another
Assessments are part of curriculum
Investigation: mathematical situation that is presented to students with the goal of exploring different aspects and arriving at summary conjectures (Huetinck & Mushin, 2008, p. 375).
When assessing student work on investigation, teachers must look for logical processes of mathematics and the connections students make with prior knowledge
More inductive than a project
Interactive Mathematics Program (IMP) poses problems of the week (POWs). POWs require students to investigate the nature of nonstandard problems.
invent some extensions or variations to the problem (related problems that can be easier/harder/same level as the original problem)
State the solution clearly and explain how they know the solution is correct and complete
Describe the steps in detail, using notes as reminders. Include work that did not work out
State the problem clear enough that someone unfamiliar with the problem can understand what it is
3 criteria included in assessment:
1. a clear mathematical objective is identified
2. the requirement to show the work leading to the solution of the problem
3. students are expected to justify what they did by discussing the results
(Huetinck & Mushin, 2008, p. 364)
rubric aims to establish a student's quality of understanding of the concepts involved
Typically, a 4-point rubric is used to indicate students' level of attainment on the particular task
give specific criteria for distinguishing between a paper that is acceptable but average and one that is exceptional and shows good understanding
External assessments - such as standardized test, performance assessment resources, and advanced placement tests - move away from relying on oversimplified evidence from a single test or test format, presents disaggregated data giving breakdowns of results in various groupings rather than single score results, and includes teachers' professional judgments about student performance
only revised every 5 to 10 years, so the evaluation score may be artificially boosted by teacher familiarity of the test
High likelihood that assessments will omit items covered in content that most students know
There is a chance that the assessments are not matched with what the teachers are actually teaching
2
Evaluation (NCTM, 1991, pp. 72-23)
Because teaching is complex, the evaluation of teaching is complex. Simplistic evaluation processes will not help teacher realize the vision of teaching mathematics described in these standards.
What teachers learn from the evaluation process is related to how the evaluation is conducted.
ALL teachers can improve their teaching of mathematics
The goal of evaluating the teaching of mathematics is to improve teaching and enhance professional growth.
NCTM Principles and Standards - 4 parts
Part 4
Steps Necessary to Achieve the Vision
Part 3
Process Standards
Representations
Connections
Communication
Reasoning and Proof
Problem Solving
Part 2
Content Standards
Data Analysis and Probability
Measurement
Geometry
Algebra
Number and Operations
Part 1
Overview of Principles
Technology
Assessment
Learning
Teaching
Curriculum
Equity
Ideally every new teacher would have a mentor teacher to guide them, but such resources are often unavailable.
Continuing Professional Development
Online Professional Development
Content Based Coaching
Higher Education
Leadership Development
Certification
State level certification - required to teach
Sets professional development requirements for continued certifcation
NBPTS - National Certification overseen by Educational Testing Service (ETS)
Case Studies
Organizations
MED
JRME
MT
MTMS
TCH
NCTM
Academic Clubs
Maybe parents can become educator leaders as well by taking role in school clubs, or academic clubs such as the maths club. Promote learning to the community as well. More educators means more accomplishments. (p.417)
Parent involement Huetinck & Mushin, 2008, p. 392-417)
Subtopic
use of technoolgy or other social media to communcate with arents. (p.404) This would enforce communitcation
Make back to school nights useful. Teachers should community as much with the parents and seek their help.
Communicating with parents will empower both students and teachers to work together in order to meet their students needs. I have witness this in my school where parents from low income backgrounds are knowledgeable about their child education and help them succeed and enforce their schools policies in their homes. (p. 400)