door Kevin Luxmore 12 jaren geleden
526
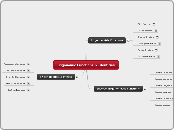
Trigonomietric Identities
Chapter 7 Project
door Kevin Luxmore 12 jaren geleden
526

Meer zoals dit
tan u = sin u / cos u
cot u = cos u / sin u
csc u = 1 / sin u
sec u = 1 / cos u
cot u = 1 / tan u
sin(-u) = -sin u
cos(-u) = cos u
tan(-u) = -tan u
csc(-u) = -csc u
sec(-u) = sec u
cot(-u) = -cot u
sin( π / 2 – u ) = cos u
cos( π / 2 – u ) = sin u
tan( π / 2 – u ) = cot u
csc( π / 2 – u ) = sec u
sec( π / 2 – u ) = csc u
cot( π / 2 – u ) = tan u
sin^2 u + cos^2 u = 1
tan^2 u + 1 = sec^2 u
cot^2 u + 1 = csc^2 u
y = sec-1 x means x = sec y
where
|x| >= 1
and
0 <= y <= π
y != π / 2
y = csc^-1 x means x = csc y
where
|x| >= 1
and
-π / 2 <= y <= π / 2
y != 0
y = cot^-1 x means x = cot y
where
-∞ < x < ∞
and
0 < y < π
y = tan^-1 x means x = tan y
where
-∞ < x < ∞
and
-π / 2 < y < π / 2
y = cos^-1 x means x = cos y
where
-1 <= x <= 1
and
0 <= y <= π
y = sin^-1 x means x = sin y
where
-1 <= x <= 1
and
-π / 2 <= y <= π / 2
csc u = 1 / y
sec u = 1 / x
cot u = x / y
tan u = y / x
cos u = x
sin u = y