Math Log #1

Week 3 and Week 4: Addition and Subtraction
How might your students' understanding of addition and subtraction progress/develop/evolve? What will you do as the teacher to help move your students toward proficiency?
My students' understanding of addition and subtraction might progress/develop/evolve by...

Using different modelling and counting strategies
Using the Student continuum of numeracy chart

For example: Counting:
Counting blocks together three times
Counting on/counting back
Counting on from the larger number

For example: Working with numbers:
Counting using the 5 or 10 anchor
Using a known fact
Using up/down over 10
Splitting up the numbers
For example:
For example:
The way the problem is structured

For example: "If you have 27 cards, how many more will you need to get 62 cards?"
"If you have 62 cards and you take away 27 cards, how many will you now have?"
As the teacher I might.... to help move my students toward proficiency.

Use different strategies to show that by adding to something makes it bigger.

For example: Using your hands
Using blocks
Using stickers
Using food

Use different strategies to show that if you take away an amount the number gets smaller

For example: Using food
Using fingers
Using blocks
Is it enough for your students to memorize addition and subtraction facts? Is it important for students to know their facts automatically?
I believe it is important for students to memorize the basic facts of addition and subtraction to help them so they can quickly come to an answer
For example: Knowing the basic equations can help students when math becomes more difficult to answer questions instead of them having to think about the simple equation first before trying to figure out the bigger part of the equation.
Having memorized some of the basics it can help compeleting equations in the future
For example: Being in the "real world" and being able to calculate your total to be sure you are not being ripped off
Can make solving equations quicker
For example: If the student automatically knows that 10 plus 10 is 20 then it will be easier for them to know that 110 plus 110 is 220 because they already know what 10 and 10 is.
What it says about addition and subtraction in the textbook...

Direct modelling

Using drawing to solve an equation

Using concrete materials
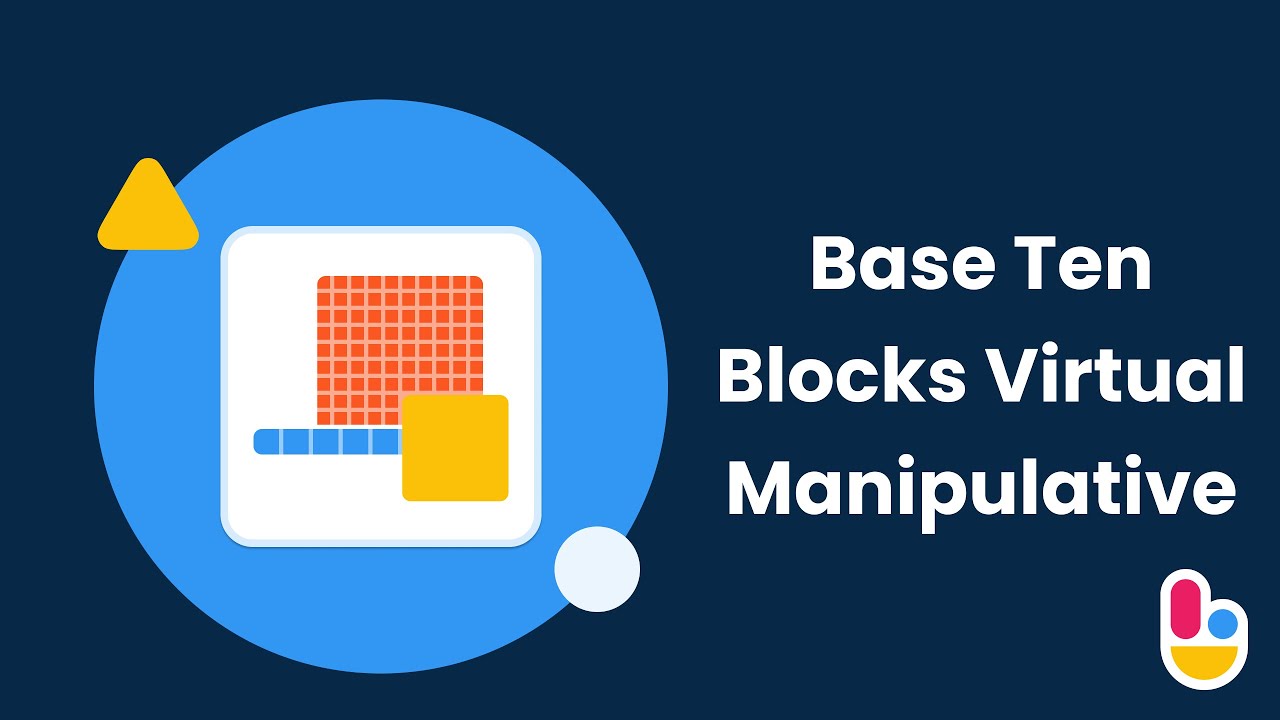
Using base ten blocks

Written supports

Charts

Stacked number lines

Number lines
Counting on and counting back
Learning about and recording jump strategies

Bar diagrams
Work well for contexts that fit a subtraction comparison situation and a part-part-whole model

Partitioning
Splitting a number into two parts

Technology

Using a calculator

Week 2: Number Sense/Place Value
What does "strong number sense' mean to you?
Strong number sense to me means that...

The student has an understanding for numbers.

They can look at a number and understand what the the quantity of the number is.

They have an understanding that there is a specific order to numbers.
How will you know if your students have strong number sense and how will you ensure they develop this?
I will know that a student has a strong number sense by...
If they are able to understand what number is which.

For example: 5 = Five
They can understand that the numbers can be shown in different ways.
For example:
They know that the numbers are ordered in a specific way.

For example: 2 comes after 1 and 3 comes after 2: but 3 does not come after 1
I will ensure they develop this by...
Using different strategies to show them how to count
For example: Counting with fingers, blocks, toys, etc...
Use different strategies to show that numbers have an order
For example: 1, 2, 3, 4, 5, 6...
Using dice
Using fingers
Using pictures
Use different strategies to show what numbers look like.
For example: Pictures, tires, dice, domino, hands, etc...
What is says about number sense in the textbook...

Counting

Being able to count in order
For example: 1, 2, 3, 4, 5...
Not 1, 4, 2, 6, 3...

Quantity
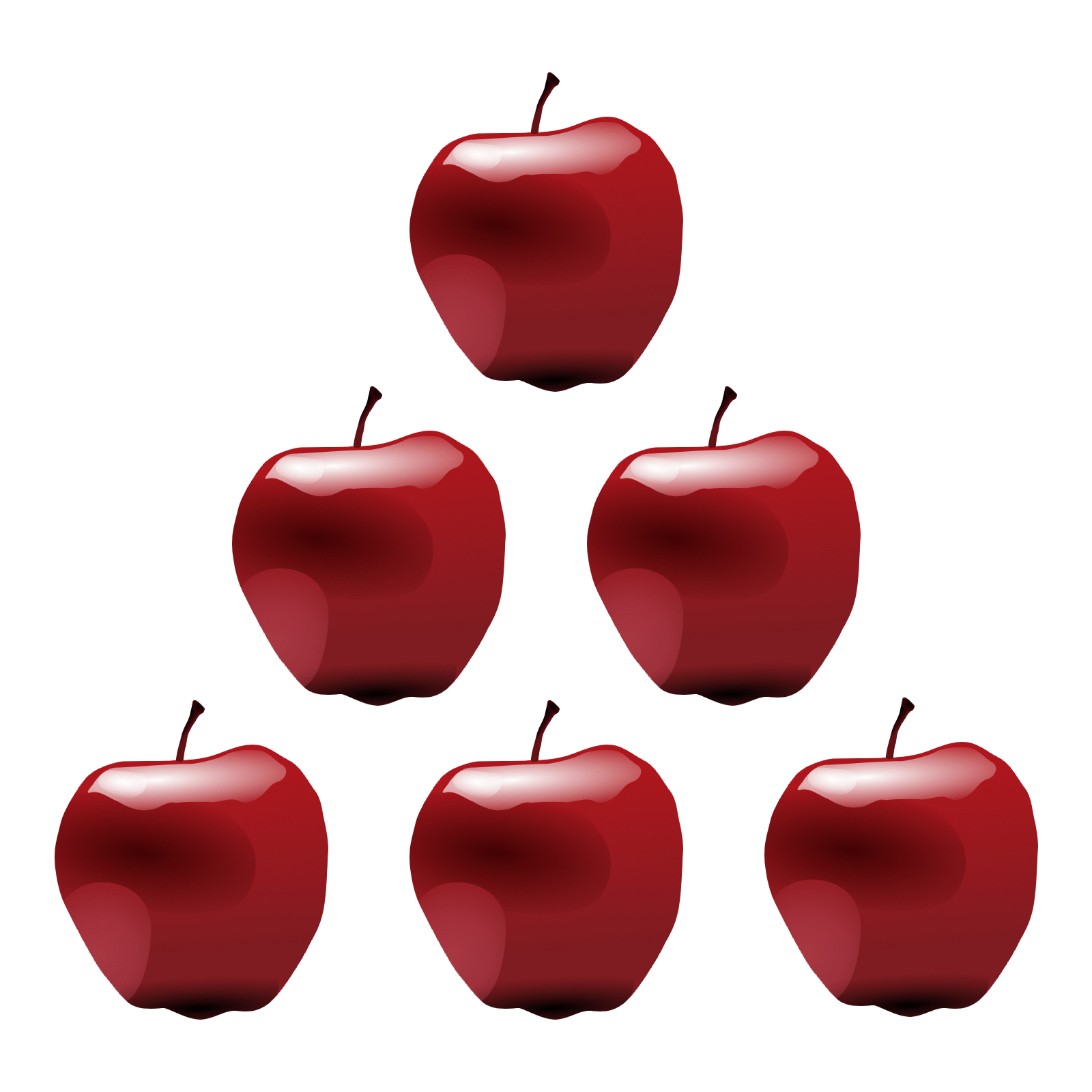
Knowing that 6 apples is 6 apples and not 4

Knowing how many

Knowing that there are 5 chocolate chip cookies on the plate

More than
Knowing that 7 is bigger than 4

Less than
Knowing that 2 is less than 8

Equal to
Knowing that 2 is 2
Developing number realtionships
Having a general understanding of number and operations as well as the ability to apply this understatement
For example: