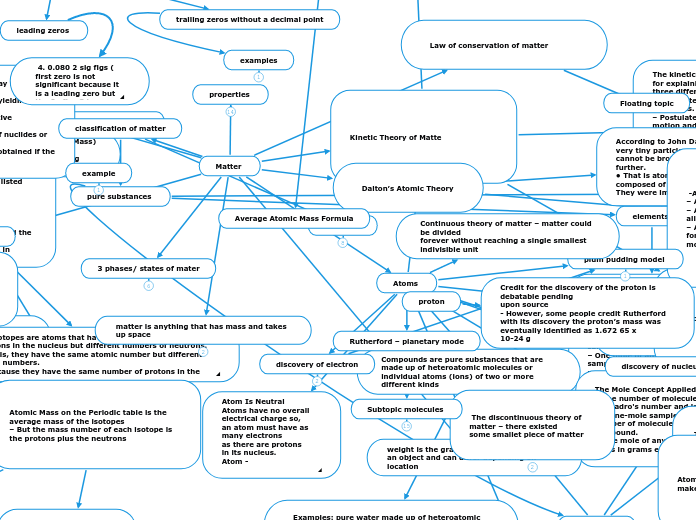
Matter
properties
atomic weight/ average atomic mass
Average Atomic Mass
In looking at the masses of the elements on the periodic
table it is evident that most values listed aren’t close to
being whole numbers
Why?
These values are actually weighted averages that represent
all the naturally occurring isotopes and the relative
abundance in which they are found in nature
Atomic Weight (Average Atomic Mass)
is the weighted average
mass of all the naturally occurring
isotopesof that element.
Average Atomic Mass
• The average atomic mass may be determined by
calculation from any analysis yielding the actual masses
of the isotopes and some relative proportion of their
presence (an actual number of nuclides or aspercentages of nuclides)
• A “rough estimate” may be obtained if the mass numbers
are utilized
classification of matter
example
pure substances
Examples: Gold (Au), Phosphorus (P4), Oxygen (O2)
elements
Elements are pure substances that are made up of homoatomic molecules or individual atoms of the same kind
Compound
Examples: H2O, C6H12O6, AgCl
Compounds are pure substances that are made up of heteroatomic molecules or individual atoms (ions) of two or more different kinds
rows
periods
Rows are called Periods
• Seven Seven periods for the seven energy levels(rings)
Formulas are used to represent compounds.
Subtopic
elements
Element is the simplest form of matter that
can not be broken down by chemical means;
One element can be changed into another
element by nuclear methods
• Are the building blocks of matter
• Currently: Type of matter composed of atoms that all have the same atomic #s (Identical atoms)
• 118 elements known today Of the 118 known only the first 98 are known to
occur naturally on earth
• Those that do not occur naturally have been artificially produced by man as synthetic products of nuclear reactions such as Einsteinium ,
Nobelium
Hydrogen (H) The lightest & the most abundant element in the universe [75%, followed by Helium 23%]
• Carbon (C) The 2 nd most abundant
element[18.5%] in human body after
Oxygen
• Oxygen (O) The most abundant element on earth crust [47% followed by Si 28%]
A symbol is assigned to each element. The symbol is based on
the name of the element and consists of one capital letter or a
capital letter followed by a lower case letter.
– Some symbols are based on the Latin or German name of the
element.
A. Calcium
2) Ca
B. Sulfur
1) S
C. Iron
3) Fe
molecules
A molecule is the smallest
particle of a pure substance that
is capable of a stable
independent existence
* Diatomic molecules contain two atoms.
• Triatomic molecules contain three atoms.
• Polyatomic molecules contain more than three atoms.
Subtopic
Subtopic
Homoatomic Molecules
• The atoms contained in homoatomic molecules areof the same kind.
Heteroatomic Molecules
• The atoms contained in heteroatomic molecules are of two or more kinds.
Periodic table
element symbol
columns
The columns are called FamiliesFamilies oror Groups
• Earlier Version had 1-8 followed by A or b
Group A elements are called Representative Elements
Group B elements are called TransitionTransition ElementsElements
• Modern Version labels the columns with 1-18Modern Version labels the columns with 1-18
Three Types of Elements
• Metals
Shiny when smooth and clean
Solid at room temperatur
• Only exception - Mercury
Good conductors of heat and electricity
Most are ductile and malleable
• Metalloids
• Nonmetals
Mixtures
Law of conservation of matter
Kinetic Theory of Matte
Ave. At. Mass = [(% x isotope mass) + (% x isotope mass) + .....]/ total %
AW(average weight)= (19.78%)10.01u)+(80.22%)(11.01u) / 100/= 198.u+883.2u/100= 10.81u
Dalton’s Atomic Theory
3 phases/ states of mater
isotopes
Isotopes of Carbon and Hydrogen
Isotopes of Hydrogen
protium deuterium tritium
11H 21H 31 H
Isotopes of Carbon
116C 12 6C 136C 146C 156C 166 C
3617Cl OR Cl-36
atomic mass
the carbon-12 atom
12 atomic mass units.
1 u = 1/12 the mass of a Carbon-12 atom.
matter is anything that has mass and takes up space
weight is the gravitational force acting on an object and can differ depending on location
scientific notation
Express use scientific calc (EXP) botton 1.8 x 10^-4 in decimal notation.
anser 0.00018
Express 4.58 x 10^6 in decimal notation.
4,580,000
On the graphing calculator, scientific notation is done with
the button. (EXP)
4.58 x 10^6 is typed 4.58x10 (EXP) 6
^
scientific notation is used to express very small or very large numbers and maintain correct number of significant figures
^
scientific notation to standard notation
Subtopic
rules for multiplication
Rule for Addition and Subtraction
Rules for Division
a x 10 ^n
Atoms
proton
Credit for the discovery of the proton is debatable pending
upon source
- However, some people credit Rutherford with its discovery the proton’s mass was eventually identified as 1.672 65 x
10-24 g
discovery of electron
Atom Is Neutral
Atoms have no overall electrical charge so,
an atom must have as many electrons
as there are protons
in its nucleus. Atom -
• Nucleus: Proton + Neutron
• Electron
^
discovery of nucleus
plum pudding model
Continuous theory of matter – matter could be divided
forever without reaching a single smallest
indivisible unit
The discontinuous theory of matter – there existed
some smallet piece of matter
neutron
Atoms are the particles that
make up molecules.
Rutherford – planetary mode
Subtopic molecules
Measurements undefined
exact number
example
Average Atomic Mass Formula
count all numbers exept
leading zeros
trailing zeros without a decimal point
examples
SI units
Subtopic
Density
Mole
A length of rope is measured to be 1834 cm. How many
meters is this?
The factor- method for solving numerical problems is a four-step
systematic approach to problem solving.
• Step 1: Write down the known or given quantity. Include both the numerical value and units of the quantity.
• Step 2: Leave some working space and set the known quantity equal to the
units of the unknown quantity.
• Step 3: Multiply the known quantity by one or more factors, such that the
units of the factor cancel the units of the known quantity and generate the
units of the unknown quantity.
• Step 4: After you generate the desired units of the unknown quantity, do
the necessary arithmetic to produce the final numerical answer.
example
1834cm(1m/100cm)=18.34m
common conversion factors
Floating topic
Floating topic
Floating topic
electron configuration
– A maximum of 2 electrons per orbital
Exceptional Electron Configurations
Noble Gas Core Electron Configurations
• Recall, the electron configuration for Na is: Na: 1s2 2s2 2p6 3s1
• We can abbreviate the electron configuration by indicating the innermost electrons with the symbol
of the preceding noble gas.
• The preceding noble gas with an atomic number less than sodium is neon, Ne. We rewrite the electron configuration:
Na: [Ne] 3s1
The periodic table can be used as a guide for electron configurations.
• The period number is the value of n.
• Groups 1A and 2A have the s-orbital filled.
• Groups 3A - 8A have the p-orbital filled.
• Groups 3B - 2B have the d-orbital filled.
• The lanthanides and actinides have the f-orbital
filled. We can use the periodic table to predict which
sublevel is being filled by a particular element.
^
Three rules—the aufbau principle, the Pauli
exclusion principle, and Hund’s rule—tell you how to find the electron configurations of atoms.
• We use a numbering system to indicate
electrons in an atom
• Its given first by a number: 1,2,3,4, etc...
dictated by the period number electron shell
• Then follows a lower case letter: s,p,d, f...
dictated by the group from the periodic table orbital type
• Then follows a superscript given over the letter: indicates number of electrons in that orbital
• First, determine how many electrons are in the
atom. Iron has 26 electrons.(nutral atom electrons and atomic number/#protons is the same)
• Arrange the energy sublevels according to
increasing energy:
–1s 2s 2p 3s 3p 4s 3d ...
• Fill each sublevel with electrons until you have
used all the electrons in the atom:
–Fe: 1s2 2s2 2p6 3s2 3p6 4s2 3d 6
• The sum of the superscripts equals the atomic
number of iron (26)
– K+
– As3+
• Write a ground state electron configuration for
these ions.
O2-
Na+
21
• Write the electron configuration of the neutral atom.
• Remove electrons from the orbital with the highest principal QN (value of n)
– Fe [Ar] 4s2 3d6
– Fe2+
• A. Fe [Ar] 4s2 3d6
• B. Fe [Ar] 3d6
• C. Fe [Ar] 4s2 3d4
• D. Fe [Ar] 4s1 3d1
An excited atom has an electron or electrons which are
not in the lowest energy state. Excited atoms are
unstable energetically. The electrons eventually fall to a
lower level. * is used to indicate an excited atom. For
example: *Li 1s2 3p1. (The ground state for Li is 1s2 2s1.)
• Write an excited electron configuration for the following
atoms.
• *Al
• *K 22
Energy Level, n # of sublevels Letter of sublevels # of orbitals per sublevel# of electrons in each orbital Total electrons inenergy level
1 1 s 1 2 2
2 2 s p
1
3
2
6 8
3 3
s
p
d
1
3
5
2
6
10
18
4 4
s
p
d
f
1
3
5
7
2
6
10
14
32
The way in which electrons are arranged around the nuclei of atoms.
Arrangement of Electrons in Atoms
SHELLS(n)
total of 7
– 1st energy level is closest to nucleus
– Contain Each energy level does not contain the same sublevels
– As the distance from the nucleus increases energy levels can hold more electrons –Therefore, they can have more
sublevels
• Four types
–s (lowest energy)
–p
–d
–f (highest energy)
ORBITALS (m1)