Basic Measurement: Perimeter, Area, Volume, and Surface Area
The purpose of this mind map is provide resources for exploring and clarifying the topic. The audience is elementary students.
area (A)
The area of a region is the amount of space inside the boundary (or perimeter) of a 2D object. Think about the number of tiles required to fill a shape with no overlapping.
area of rectangles
For all rectangles: A=wl (Area = Width x Length)
area of parallelograms
Area of parallelogram: A=bh (Area=base x height)
area of triangles
Area of triangle: A=(1/2)bh (Area = one half Base x Height)
a
area of trapezoid
Area of trapezoid: A=(1/2)(a+b)hwhere a and b are the lengths of the two bases and h is the height of the trapezoid
area of circles
Area of a circle: A=πr2 where r is the radius of the circle.
perimeter (P)
The perimeter of a region is the length of its boundary, just the outer edge of a 2D shape (or region).
Perimeter of polygons
To find the perimeter of a polygon add the lengths of all of its sides.For example: if I have a square with side lengths of 3 ft., I will add 3+3+3+3= 12 ft.

perimeter of a circle
The perimeter of a circle is called its circumference. It is found with the formula C=2πr where C is the circumference and r is the radius of the circle.
The Measurement Process

Four steps
1. choose an attribute
Choose the attribute to be measured: length, area, volume, capacity, temperature, time, or weight.
2. select a unit
2. Select an appropriate unit of measurement.The unit of measure will change based on what you are measuring. Possibilities for measuring length include, but are not limited to: inches, feet, miles, centimeters, meters, kilometersFor weight you could use: ounces, pounds, tons, milligrams, grams, or kilograms.Remember to chose a unit that makes sense for what you are measuring.
3. make a comparison
3. Use a measurement device to compare the object to the unit of measurement.A measurement device is a tool or instrument used to measure: ruler, tape measure, meter stick, gauge, scale, thermometer, etc.
4. express as number of units
4. Express the measurement as the number of units used in the comparison.

Units

International System of Units

SI prefixes
metric units of area
metric units of volume

U.S. Customary System of Units

U.S. units of length

U.S. units of area
Units of area will be the unit of measurement squared, for example the area of a rectangle measured in inches will be given as ___ square inches (or in.2). This is because we are considering two dimensions of the object: length and width. Think 2D therefore squared.
U.S. units of volume
Units of volume will be the unit of measurement cubed, for example the volume of a rectangular prism measured in inches will be given as ___ cubic inches (or in.3).This is because there are three measurements used to calculate volume: length, width, and height. We are considering three dimensions of the object, think 3D therefore cubed.

U.S. units of capacity

capacity conversions
Pythagorean Math
Uses

slant height
The slant height of a cone can be determined by thinking about a triangle where one leg is the radius of the base and the other leg is the vertical height of the cone, the slant height would be the hypotenuse.Thus: Slant Height2=h2+r2where h is the vertical height and r is the radius of the cone

unknown side
If two sides of a right triangle are known, then the length of any unknown side can be found using the theorem a2 + b2 = c2
Right triangle
If a2 + b2 = c2 then it IS a right triangle.If a2 + b2 ≠ c2then it IS NOT a right triangle.

theorem
a2 + b2 = c2The sum of the areas of squares on the legs of a right triangle is equal to the square on the hypotenuse.
volume (V)
Volume is the amount of space enclosed within the boundary of a 3D shape (or object).
volume of a box
The volume of a rectangular box (or prism) is found by multiplying length x width x height.Use the formula: V=Bh where B is the area of the base and h is the height.Note: to find the volume of a complicated prism, decompose it into simpler shapes (watch the video to see a demonstration of this)

volume of a cylinder
Volume of a cylinder: V=Bh where B is the area of the base and h is the height.Notes: the base of a cylinder is a circlethis same formula is used to find the volume of right, oblique, or general cylinders!

volume of a pyramid or cone
Volume of pyramid or cone: V=(1/3)Bh where B is the area of the base and h is the height.Notes: a pyramid will fill (1/3) of a rectangular prism with the same base and heighta cone will fill (1/3) of a cylinder with the same base and height

volume of a sphere
Volume of a sphere: V=(4/3)πr3where r is the radius of the sphereNote: the volume of a sphere takes up (2/3) the volume of a cylinder of the same radius and diameter height
surface area (SA)
Surface Area (SA or S) is the boundary of a space figure. A space figure is an object that is represented in three dimensions (3D).Total surface area is the sum of the base area and lateral area (or sides) of a space figure.Think about a jacket or cover that would enclose the entire shape.
SA of right prism
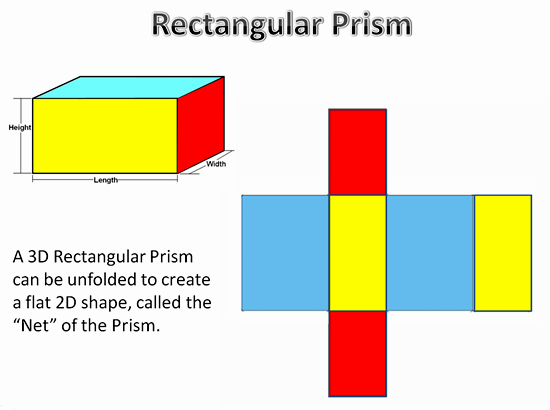
Surface Area of Right Prism or Cylinder:SA=2B+phLet h be the height, and B be the areas of both bases, and p be the perimeter of each base.Note: if you unfold a prism you have a collection of 2D shapes called the net of the prism
a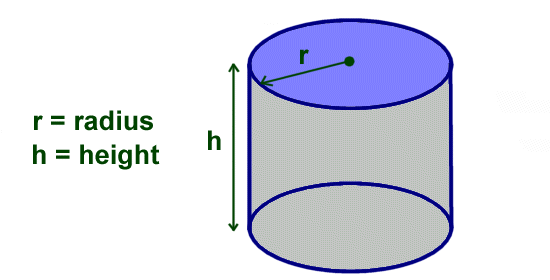
SA of a cylinder
Surface Area of Right Prism or Cylinder:SA=2B+phLet h be the height, and B be the areas of both bases, and p be the perimeter of each base.Note: if you unroll the side of a cylinder you have a rectangle

SA of right rectangular pyramid
Surface Area of a Right Regular Pyramid:SA=B+(1/2)psLet s be the slant height, and B be the base area, and p be the perimeter of the base.Note: to determine slant height use the Pythagorean Theorem: s= √(x2-y2) given x is the height of the pyramid and y is the (1/2) the length of one side of the base of the pyramid. When thinking about this triangle let s be the hypotenuse, and x and y be the two legs.

SA of a cone
Surface Area of a Right Circular Cone:SA=πr2+πrsLet s be the slant height and r be the radius of the base.Note: to determine slant height use the Pythagorean Theorem: s= √(x2-y2) given x is the height of the cone and y is the radius of the cone. When thinking about this triangle let s be the hypotenuse, and x and y be the two legs.
SA of a shpere
Surface Area of a Sphere:SA=4πr2Let r be the radius of the sphere.
