作者:Justin Charno 2 年以前
373
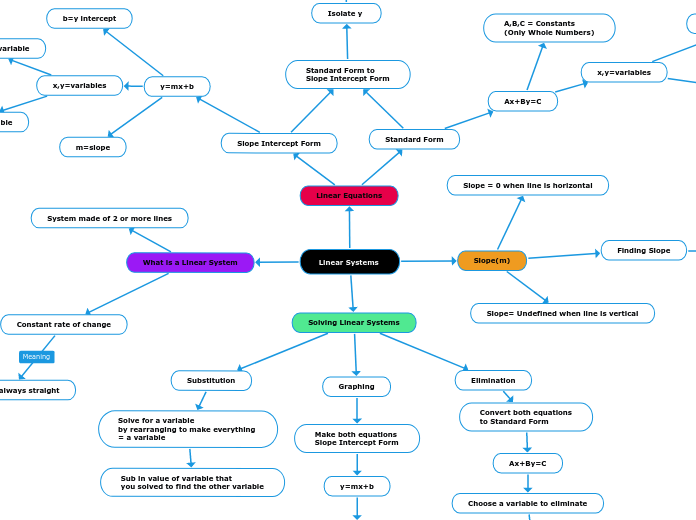
Linear Systems
The study of linear systems involves understanding equations that depict straight lines with a constant rate of change. A fundamental representation is the slope-intercept form, y=mx+b, where '
作者:Justin Charno 2 年以前
373

更多类似内容
y2-y1 _____ x2-x1
Isolate y
Move everything to the right side of the = sign except y
Numbers sign changes when moved over = sign
2x+y=8
y=-2x+8
A,B,C = Constants (Only Whole Numbers)
x,y=variables
x = independent variable
y = dependent variable
b=y intercept
m=slope
Ax+By=C
Choose a variable to eliminate
Multiply both equations by a number to make the coefficients same or opposite
Add/Subtract the equations to eliminate the chosen variable
Sub in value of variable that you solved to find the other variable
y=mx+b
Start each line at y-int(b)
Use the equation of the slope to draw the lines
If slope is positive, line increases from left-right
If slope is negative, line decreases from left-right
Sub in value of variable that you solved to find the other variable