af Kumar Perumal 4 år siden
881
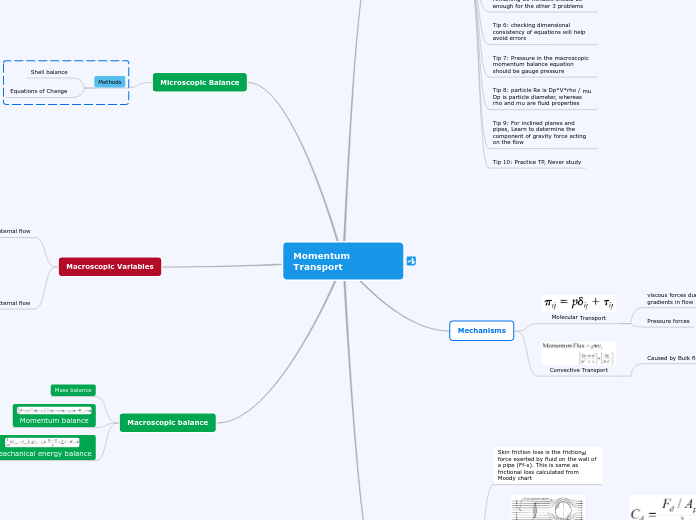
Momentum Transport
The text discusses various aspects of fluid dynamics, focusing on flow in different geometries such as pipes, planes, plates, and annular pipes. It delves into the concept of momentum transport, explaining that it comprises both molecular and convective momentum transport.