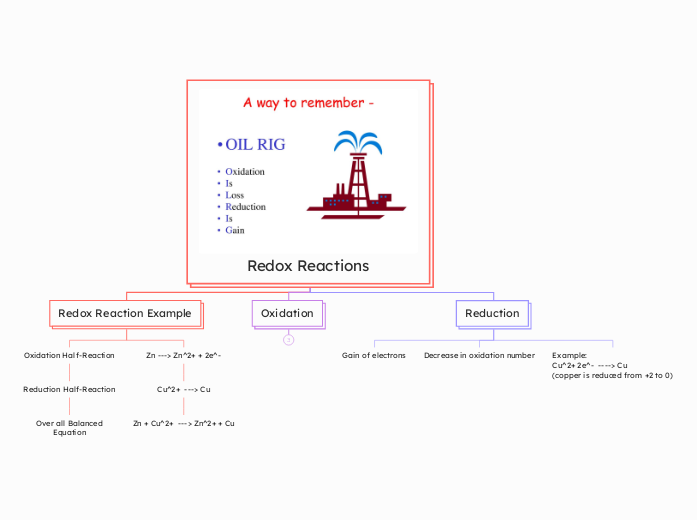
von Mainal Lodhra Vor 6 Jahren
772
Math Summative
In the study of analytic geometry, understanding key concepts such as the y-intercept and rate of change is essential. The y-intercept is the point where a line touches the y-axis and can be found by setting x to zero in the equation of the line.