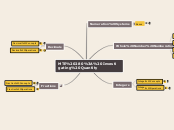
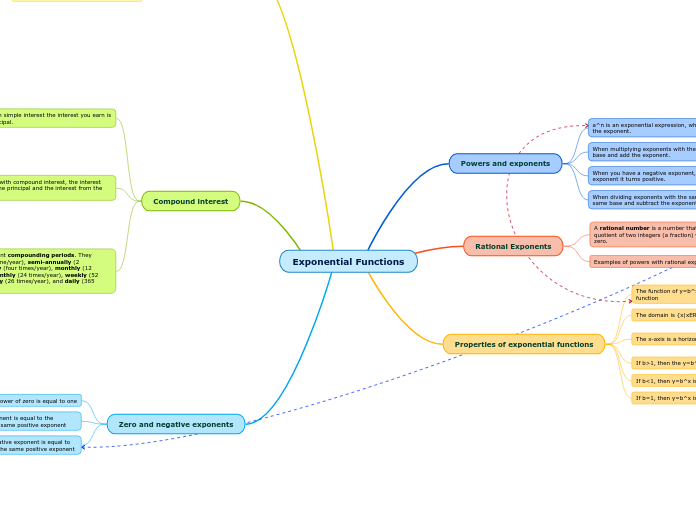
MTE 280: Investigating Quantity
Fractions
Fractional size is relative!
- Fraction language: The number of parts that makes up a whole determines the name of the fractional parts or share. Ex --> halves, thirds, fourths
- Top number counts (numerator)
- Bottom number tells what is being counted (denominator)
Mixed Number: whole number and a fraction (ex: 1 3/4)
Applications of Fractions:
Models for Addition and Subtraction:
- Discrete: objects (set model)
- Continuous: number line (linear)
- Take Away: set/area model
- Comparison: linear/area model
- Missing Addend
Fraction Operations
Fraction Addition
You may use any of the three models to add and subtract fractions.
For example, let's add 5/9+1/3 using the set model.
Draw nine circles or any other type of unit. Then, color in five to show five ninths. We must add 1/3 in now, so in order to do that we need to break up the nine units in three groups. We find that there is three in each group which represents one third. Use a different color or marking, and mark the three units that shows 1/3 after the five ninths. If we add up all the marked/colored units, you will see eights colored units out of nine totals parts to get a answer of 8/9.
Fraction Subtraction
For example, let's solve 3/4-1/8 using the linear model. First draw 3/4 and mark in on the number line between 0 and 1, then split the number line in eighths and mark it it starting with 1/8 and then 2/8 and so on. When you subtract 1/8 from 3/4 you get 5/8, because it is 1/8 away from 3/4, so the answer is 5/8.
Fraction Multiplication
For multiplication, an efficient way to represent fractions uses the area model of multiplication which is slightly different than subtraction and addition.
For example, lets solve 2/3 times 4/5.
This literally means there are 2/3 rows of 4/5.
First draw a unit square and cut it into five pieces and color in four. Label 4/5 at the top of the square. Then split the square in thirds to make three rows. Since we need two-thirds of the rows, color in or mark the two of the three rows we made. Then count how many in the colored in pieces there are, and you should get 8, and count how many pieces there are overall in the square and you should get 15, so the answer is 8/15.
Fraction Division
An easy way to show fraction division is using rulers, which is similar to the measurement model because we are finding how many groups there are by using rulers, which are like number lines.
For example, lets solve 2 1/2 divided by 3/8.
This literally means how many 3/8 are there in 2 1/2.
To solve, first mark 2 1/2 on the ruler. Then start at zero and find three eights on the ruler and jump to it. Label this as 1 jump. Then, keep going by moving three eighths (if you need help count the tick marks), until you reach or go past 2 1/2. In this problem, you will end up going past 2 1/2.
Count the number of jumps which is 6 jumps, and count the number of pieces in the jump, and it turns out that there is 3 pieces. 2 and 1/12 lies on the second piece out of three pieces. So the answer to 2 1/2 divided by three eighths is 6 and 2/3.
Link
(The link will take you to the home page, but you will see the web pages for addition, subtraction, multiplying and dividing which you can click on. They call the linear model, a line model, and the area model and circle model, and unfortunately there is no set model, but it is a good link for seeing the area and line model. )
Fraction Concepts
Fraction Concepts
A proper fraction represents a number between zero and one. An improper fraction is always greater than one because the numerator is bigger than the denominator.
- The "wholes" have to be the same size when comparing fractions, or in other words the fraction size has to be relative.
- Fractions have to have equivalent sized fractional pieces. So for example when drawing a pizza to represent different fractional pieces, each piece has to be the same size.
Counting Fractional Parts: Iteration
In the fraction 1/4, the top number is called the numerator and it tells us how many, and the button number is called the denominator and it tells us the number of pieces. So 1/4, is a count of one part called fourths.
Fraction Misconceptions
- Thinking of a numerator and a denominator as separate and not as a single value
- Not recognizing the equal parts
- thinking that fraction 1/5 is smaller than 1/10 for example, just because it has a smaller denominator
- Using the rules from whole numbers to compute
ex.) a fraction times a fraction is smaller
The link attached introduces all of the three models, area, set, and linear/length model. Note that in the link, when the examples/manipulatives you can use to do the set model is discussed, there are more examples that is listed on the website. For the set model, you may use any object, even stars or triangles.
Teacher should be familiar with how to represent any fraction using any of the three models.
Equivalent Fractions
You can use any of the 3 models to represent equivalent fractions.
For example, show 2/6=1/3. For the area model, you can draw a rectangle, and first split it into six even parts and color in two parts to represent 2/6. Then split the rectangle in thirds. You will see that 2/6 is equivalent to 1/3 in the drawing.
For the linear model, you may draw a number line and mark 2/6, and split the number line in sixths. Then think how many sixths are in one third by drawing thirds on the number line. After marking the numbers you should find that two sixths is the same as 1/3 on the number line, just as four sixths is the same as 2/3.
For the set model, one way you can show equivalent fractions is to draw six units, such as circles, and color in two of them to show two sixths. Then, split the units in thirds so that there is two units in each of the three groups. You can use a different color or circle them and you will visually see that one third is also two sixths.
**For mixed numbers, you do not have to change them to an improper fraction to represent them using the three models. Just be sure to show that the model is showing either the mixed number or the improper fraction, because for example, visually representing 1 2/3 is sometimes different than showing 5/3
Estimating and Comparing Fractions
To estimate fractions in terms of how much bigger or smaller there are compared to other fractions, and to find out what number the fraction is close to we use benchmarks.
Bench marks are 0,1/2,1
For example, where is four ninths close too?
One way to figure this out is to draw out nine units in the set model and color four in. Where does this drawing look close too? You can see that it looks close to 1/2, so four ninths is going to be slightly less that 1/2.
Another example to compare fractions using benchmarks:
3/8 or 4/7? Which is bigger?
4/7 is greater because it is bigger than 1/2, and 3/8 is less than one half.
Decimals
Decimal Operations
Addition and Subtraction
You can add decimals essentially with the same traditional algorithm for addition of integers. Pictorially, you can use the base 10 blocks, converting from units to longs and longs to flats, and flats to cubes as necessary. Subtraction can be done with the blocks and pictorially as well, crossing out flats, longs and units as needed.
Multiplication
Whole Numbers by decimals
When multiplying whole numbers by decimals, you simply create however many whole groups of the decimal and combine them. This method is helpful when using pictorial representations or base ten blocks.
3 x 5.2= 1.56 llllloo llllloo llllloo = 1 whole and llllloo
Decimal by whole number
In this situation, you would draw out the whole number and then divide it into parts, shade the decimal representation and then add them together.
Decimal by Decimal
These are easier when using a grid. One grid represents a whole.
.5x.8=.4
You begin by shading in 5 rows or longs/tenths and then 8 columns or tenths in the other direction. Then, you count the units that are shaded in by both decimals and that is your product.
Division:
When you are doing division, you always have to determine whether partition model or measurement model is going to give you the correct answer.
Whole by decimal:
For this type of division, you have to use measurement model because you cannot divide a whole number into groups that are not wholes. For example,
2/.8=2.5 groups
You cannot create 8 tenths of a group and therefore, you know that each group needs to have 8 tenths in it. So, you end up with 2 and 1/2 groups of 8 tenths.
Decimal by Whole
In this case, you have to use partition model because you cannot break a decimal into groups of whole numbers. You do not even have a whole number to begin with. You know then that you need to divide the decimal into a certain number of groups.
For example: .5/2=.25 in both groups
Decimal by decimal
You have to use measurement for these problems for the same reason as before; you can not have tenths or hundredths of a group. These are often easier if you use base 10 blocks to divide.
.6/.2=3 groups
In both cases of the measurement problems, the quotient ends up being larger than the dividend. This is because the divisor is dividing the dividend into groups that contain less than 1 and therefore, more groups are needed to use the entire dividend.
Decimal Concepts
Base 10 Block Representation:
Cube= 10 = tens
Flat= 1 = ones
Long = 1/10 =.1 = tenths
Units= 1/100 = .01 = hundredths
Place Value:
The place values are mirrored across the ones place, meaning that one step above the ones place is the tens place and then one step below would be the tenths place. They can also be represented as like: tenths= (10)^-1
Words vs. Standard form:
509.34 = five hundred nine and thirty-four hundredths
When comparing fractions, 3.4> 3.21 because 40 hundredths are more hundredths than 21 hundredths.
Expanded form:
This is the same for decimals as it is for integers in the set up, except decimals have negative exponents. For example,
3.44 = 3(10)^1 + 4(10)^-1 + 4(10)^-2
Decimals as fractions:
When you are converting decimals to fractions, .2 for example would be represented as 2/10 because one tenth is 1/10 and you have 2 tenths.
Fractions as Decimals:
There are two types of fractions as decimals: Terminating and nonterminating. Terminating decimals are decimals that have factors that are factors of 10. They have a distinct end to the number. Nonterminating decimals have a at least one prime factor that is not a factor of ten.
2/3= .6 (repeating) is a nonterminating decimal.
1/4 = .25 is a terminating decimal
Integers
Integer Operations
When completing any operation with integers, if you are missing a number that you need (positive or negative), you must add a zero pair. For example, -4 - 1 requires you to take away one negative when using the chip method (or charged field). You have 4 red (negative) chips, but you cannot take away a positive from this, so you add two chips, one positive and one negative. Then, you can take away the positive and realize that you are left with -5.
Positives/Negatives:
- Addition: when adding integers, two negatives equals and negative and two positives equals a positive.
- Subtraction: When subtracting, two negatives equals a positive. Two positives also equals a positive.
- Multiplication: When multiplying, two negatives equals a positive and two positives equals a positive.
- Division: For division, you can use the pattern strategy as well, but you can use inverse operations to use multiplication facts instead of division.
Pattern Method:
The pattern method helps you to find what your answer is by creating a pattern.
ex: (-3) x (4) = ?
3 x 4 = 12
2 x 4 = 8
1 x 4 = 4
0 x 4 = 0
-1 x 4 = -4
-2 x 4 = -8
-3 x 4 = -12
By creating a pattern with the problems you do know, you can find the answer to your problem.
Integer Concepts
Number Lines:
- Number lines are great tools to use when working with integers because integers include negative numbers, as well. For example, you could show the temperature starts at 20 degrees and then drops down to -4 degrees. The number line is a perfect representation of how much change has occurred between the two numbers.
Chip Method:
- The Chip Method is used to show numbers that are positive and negative. Negative numbers are used represented through a red (or colored in) chip. The positive numbers are represented through a white (or open) chip.
*yellow chip will represent a positive integer and a red chip will represent a negative integer*
Absolute Value:
- The absolute value of a number is the quantity or worth of a number. It doesn't matter if the number itself is positive or negative, the absolute value is always positive. For example, the absolute value of -100 is 100. It is shown by using brackets, ex: |-100|
Whole Number Numberations
Is the four basic operations on
whole numbers which are addition, subtraction; multiplication and division.
Number Theory
Factors:
- Factors are the numbers that a number is divisible by.
- Factor Rainbows are used as a visual aid to show which numbers are multiplied together to get the final product. The numbers that get multiplied together are the factors.
Prime and Composite Numbers:
- Prime numbers are numbers that are only divisible by one and itself and are two different factors. For example, the only factors of 7 are 1 and 7.
- Composite Numbers are numbers that have more than 2 factors. For example, the factors of 8 are: 1,2,4,and 8.
Divisibility Tests:
- Divisibility tests are used to help determine whether or not a number is a factor of another number. They are important because they save time on division of big numbers and help you to find the factors of a number.
Divisibility Test Rules
2: Ones digit is 0,2,4,6, or 8
3: Sum of the digits is divisible by 3
4: The number formed by the last 2 digits is divisible by 4
5: One digit is 0 or 5.
6: The number is even and the sum of it's digits is divisible by 3.
8: The number formed by the last 3 digits is divisible by 8.
9: The sum of the digits is divisible by 9.
10: The ones digit is 0.
Fact Families
What two important ideas must children know about fact families?
Inverse Operations and Commutative Property.
Example: 3, 5, 15
3 X 5 = 15
5 X 3 = 15
15 / 3 = 5
15 / 5 = 3
Algorithms and Strategies
Algorithms
Addition
Traditional/Standard Algorithm of Addition: Beginning on the right and moving left, you add first the ones place values, regrouping to the tens place as needed, and then onto the tens, hundreds, thousands, etc. until you have your final sum.
48
+31
79
Partial Sums: Adding from the left to the right, you begin by adding the tens places together and writing that number below the bar. Then, you add the ones place together and place that number underneath the tens sum. Then you add those numbers together to find the final sum.
48
+31
70
+ 9
79
Expanded Notation:
Each number is broken down into expanded form, lining up the place values and adding simply within each place value position from there. Beginning with the ones places, you add, regrouping to the tens place as needed and then continuing onto the tens places.
40 + 8
+30 + 1
70 + 9 = 79
Subtraction
Traditional Algorithm of Subtraction:
Moving from the right to the left, you start by subtracting the ones place, regrouping with the tens place if needed. Then you move along to the tens, hundreds, thousands, etc. places subtracting and regrouping as you go until you have your final figure.
48
-31
17 fewer figures
Equal Addition Algorithm:
Adding the same addend to two numbers that are being subtracted does not change the final difference so in this algorithm, you add the same number to the two numbers you are subtracting. Then subtract using the traditional algorithm.
56 +2 = 58
-18 +2 = -20
38
Multiplication
Traditional Algorithm of Multiplication:
You begin with the ones place value of the second number being multiplied. Multiply that with the ones place digit of the first number, the one above it, and then recording the ones place digit in the ones place and regrouping the tens place into the tens value of the first number. Then multiply the ones place digit of the second number with the tens place digit of the first number, adding any regrouped tens place. Record this number in front of the recorded ones digit. Then move on to the tens place digit of the second number. Multiply it by the ones place digit of the first number regrouping as necessary. When recording the number, it has to be considered that the ones place digit was being multiplied by the tens place value so therefore, the number would be a multiple of ten. The then with the tens place digit of the first number, adding any regrouped tens value. Put this number in the tens and hundreds place as you are multiplying two multiples of ten. The then with the tens place digit of the first number, adding any regrouped tens value. Put this number in the tens and hundreds place as you are multiplying two multiples of ten. Add the two numbers found from the products together and that will be your final product.
1
42
x16
252
+420
672
4(367)= 4(3x10^2 + 6x10 + 7)
Lattice Algorithm:
http://sierra.nmsu.edu/morandi/coursematerials/graphics/LatticeMultiplication.gif
This is not something I can create an example for just typing. Please refer to the video I have posted with this. But with this algorithm, you multiply all the single digits and save the addition until the very end. The link above is a picture of an example.
Strategies
Open Line
<----48----58----68----78---->
>+10 >+10 >+10 >+10
Compensation
48+33
+2
50+33=83
83-2=81
Decomposition
48+33
>40 & 8
>2, 1 & 30
2+8=10
40+30=70
70+10=80
80+1=81
Regrouping: When using regrouping, students will regroup numbers into different numbers that they can easily add, subtract, multiply, or divide.
ex: 425+176
425
+ 176
------------
500
90
+ 11
-----------
500
100
+ 1
----------
601
Subtraction Comparison: When using subtraction comparison, students will break the two numbers up into numbers that can be easily subtracted.
ex: 60-->40 and 21
42-->40 and 2
40-40=0, 21-2=19
Open Number Line: When using an open number line, students can start at any place on the line that they want and then move or jump to whatever place they need to get to.
ex: 61-42:
-Number line will start at 61. One jump backwards (-40), gets you to 21. Another jump backwards (-2), gets you to 19.
*Note: Base Ten Blocks are a strategy as well
Properties and Models
Addition
Discrete: characterized by the combining of two sets of discrete objects
Continuous: characterized by the combining of two continuous quantities (time, distance)
Properties of Addition
Closure: add any 2 whole numbers & the sum is a whole number
Commutative: changing the order of the addends will result in the same sum
Associative: adding 3 or more numbers, the grouping of the numbers will not change the sum
Identity: adding zero to a number will not change the original number
Subtraction
Take Away: taking away a certain amount from the initial quantity
Missing Addend: determine what quantity needs to be added to reach the target
Comparison: comparing two quantities to determine how much larger or smaller one is
Linear: Number line using arrows to show change
Properties of Subtraction
None of the properties apply, can be proven using examples
Multiplication
Repeated Addition (SET): repeatedly adding a quantity of objects a specified number of times
Area Model: using two sides to find the total area inside a shape
Repeated Addition (LINEAR): repeatedly adding quantity of continuous quantities (such as time, distance, ect.) a specified number.
Properties of Multiplication
Closure: multiplying two whole numbers will result in a product that is a whole number
Commutative: changing the order of the numbers will result in the same product
Associative: multiplying 3 or more numbers, the grouping of the numbers will not change the product
Identity: multiplying the original number by 1 will result in the same number
Distributive: distribute the outside number and multiply it by the two numbers inside the parenthesis to solve
Zero Property: multiplying the number by 0 will always equal 0
Division
Partition (Sharing): given a certain quantity and breaking that into a specific number of groups, finding how many are in each group
Measurement: using a given quantity to create groups of a specified size
Properties of Division
None of the properties are true
Numeration Systems
Hindu-Arabic: All numerals is constructed from the ten digits; place value is based on powers of 10
1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11...20...30
Egyptian: Uses tally marks and a grouping system for numerals over 10 using Egyptian symbols
Roman Numerals: Uses symbols to represent numerals for 1, 5, 10, 50, 100, 500, and 1000; can be written using an addictive property and a subtractive property
- l, ll, lll, lV, V, Vl, Vll, Vlll, lX, X
Tally: Is a quick way of keeping track of numbers in groups of five. One vertical line is made for each of the first four numbers.
Bases
1 block = 1 unit
10 units = 1 long
10 longs or 100 units = 1 flat
1000 units or 6 flats = 1 cube
Base Two: is the "binary system" where the only two digits are 0 and 1.
1, 10, 11, 100, 101, 110, 111, 1000...
Base Five: is a "one-hand system" where 10 represents one hand with zero fingers, and the pattern continues.
1, 2, 3, 4, 10, 11, 12, 13, 14, 20...24...30...
Base Ten: is the "decimal system" or "deanery system" where each digit in a position of a number that can have an integer value ranging from 0 to 9 (10 possibilities).
0, 1, 2, 3, 4, 5, 6, 7, 8, 9 . . .
Base Twelve: is the "duodecimal system" where two new symbols are needed to represent the two new numerals.
1, 2, 3, 4, 5, 6, 7, 8, 9, X, E, 10...19, 1X, 1E, 20...30...
Example:
Given base: Base of 5
Given standard form: 3104
Draw the model: We would write out and solve what our standard form first to know how much of cube, flat, long, and units we would need . . .
(1) So for 3104, we begin as:
5^3 5^2 5^1 5^0
3 1 0 4
125 25 5 1
(2) Concluding from our answers: 3 Cubes, 1 flat, None, 4 units is what we draw out.
*TIP: If there is a zero, you can completely exclude it but the exponents need to be correct.
Word Form: Three one zero four in base five.
Expansion Form: 3(5)^3+1(5)^2+0(5)^1+4(5)^0
What's the underlined digit? 3(5)^3 = 375(Base of ten)
Finding the value form of the expanded form: 375(Base of 10)+25+0+4 = 404(Base of 10)