Definite Integrals
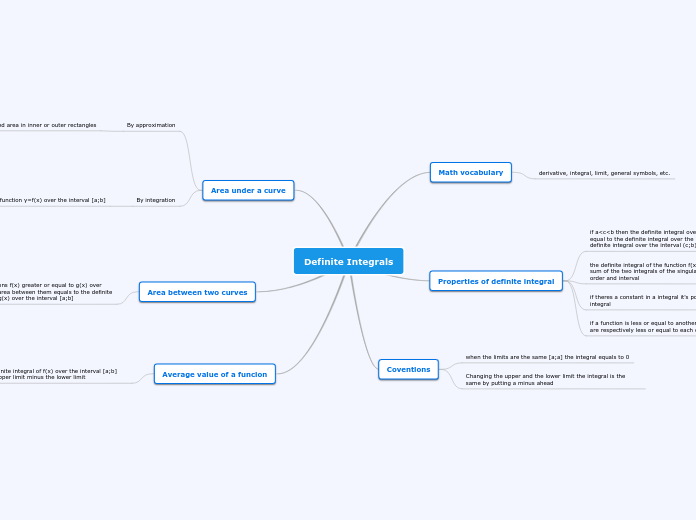
Average value of a funcion
equals to the definite integral of f(x) over the interval [a;b] diivided by the upper limit minus the lower limit
Area between two curves
If you have two functions f(x) greater or equal to g(x) over the interval [a;b] the area between them equals to the definite integral of f(x) minus g(x) over the interval [a;b]
Area under a curve
By integration
definite integral of the function y=f(x) over the interval [a;b]
Somtitimes positive sometimes negative
The limits aren't given
you need to find the intersections with the x axis
The function is negative in the interval
you need to put an absolute value at the start or you need to change the sign at the end
The function is not given
you need to find the equation of the function
The solution of the definite integral is the antiderivative of f(x) over the interval [a;b]. Then substitute the upper limit (b) into the integral and subtract the value given by substituting the lower limit (a) into the integral
By approximation
divided area in inner or outer rectangles
Coventions
Changing the upper and the lower limit the integral is the same by putting a minus ahead
when the limits are the same [a;a] the integral equals to 0
Properties of definite integral
if a function is less or equal to another one then the integrals are respectively less or equal to each other like the functions
if theres a constant in a integral it's possible to take it out the integral
the definite integral of the function f(x) plus g(x) equals to the sum of the two integrals of the singular functions in the same order and interval
if a
Math vocabulary
derivative, integral, limit, general symbols, etc.