exponential and logarithmic functions

Plus de détails

VM266 1418

par John Krause

key terms of term # 3

par nadra imtiaz

Economic Problem

par Jorge Otavo

Cranial Nerves

par Nathan Cornell

exponential and logarithmic functions

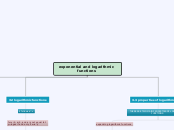
3.3 properties of logarithmic functions

THERE ARE TWO MAIN PROPERTIES OF LOGARITHMIC FUNCTIONS

condensing logarithmic functions

the 3 properties of condensing:

power property

n logb mn = logb m^n

example: 3 log6 5= log6 125

logb m - logb n = logb m/n

example: ln (x^2+5)-x= ln x+5/x

logb m + logb n = logb mn

example: log 8 + log x + 4 log y= log(8xy^4)

expanding logarithmic functions

the 3 properties of expanding:

power prperty

logb m^ n = n logb m

example: log6 125= 3 log6 5

quotient property

logb m/n = logb m - logb n

example: ln x+5/x= ln (x^2+5)-x

product property

logb mn = logb m + logb n

example: log(8xy^4)= log 8 + log x + 4 log y

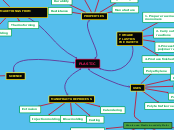
3.2 logarithmic functions

it follows a^x

for x>0, a>0, and a is not equal to 1 y=logax if and only if x=a^y

fro example: log base 3 of 9 is equivalent to 3 to the power of 2

THERE ARE THREE TYPES OF LOGARITHMIC FUNCTIONS:

to the base e

also called natural

witten as ln x

to the base 10

also called common

written as log to the x

to any base except 10

written as logd to the x

3.1 exponential functions

the exponential function f with base a is denoted by f(x)=a^x

f(x)=a*b^2, where a is a non zero, b is positive, and b is not equal to 1. the constant a is the initial value and b is the base

THERE ARE TWO TYPES OF EXPONENTIAL FUNCTIONS:

exponential decay

a>0 and b<0

exponential growth

f(x)=a*b^x

a>0 and b>0