par John Kelabu Il y a 4 années
561
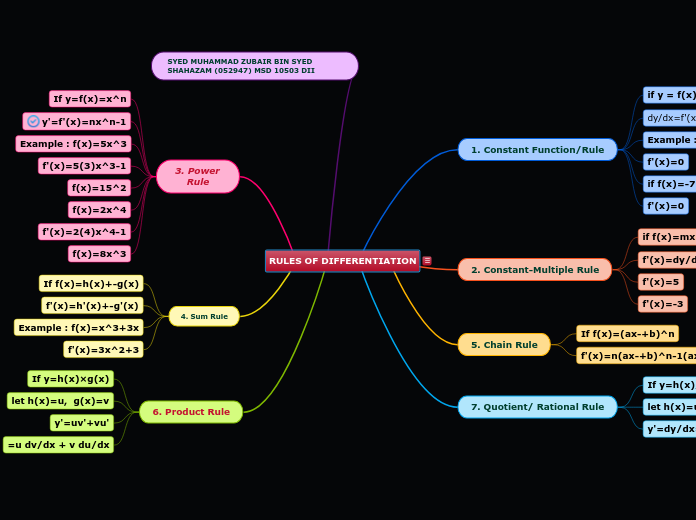
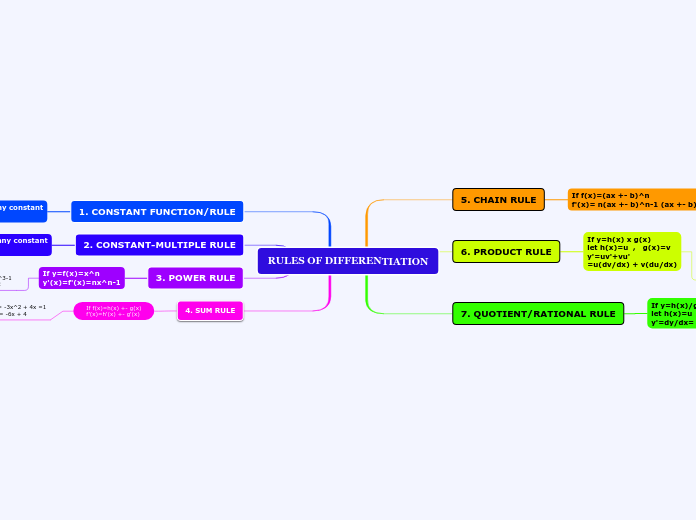
RULES OF DIFFERENTIATION
The document explains various rules of differentiation used in calculus. It covers the Quotient Rule, which is applied when differentiating a function that is the ratio of two other functions.