Chapters 3 and 5

More like this

graphics-Charity box

by Gabriella Charlesworth

vet clin path - liver & muscle

by cecle coulon

Emulsfier

by Ang Chia Kee

MUSCLES OF THE HUMAN BODY

by Derryn Pert

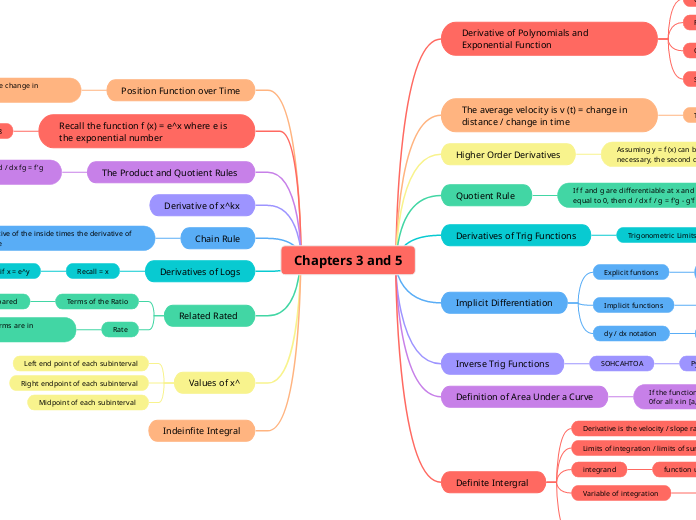
Chapters 3 and 5

Indeinfite Integral

Values of x^

Midpoint of each subinterval

Right endpoint of each subinterval

Left end point of each subinterval

Related Rated

Rate

A special ratio in which the two terms are in different untis

Terms of the Ratio

The numbers or measurements being compared

Derivatives of Logs

Recall = x

y = ln x if and only if x = e^y

The domain of ln x (0,infinity)

Chain Rule

the derivative of the inside times the derivative of the outside

Substitute u for g (x)

Derivative of x^kx

The Product and Quotient Rules

If f and g are differentiable at x, then d / dx fg = f'g = g'f

The derivative of the First times the Second plus the derivative of the Second times the First

Recall the function f (x) = e^x where e is the exponential number

e = 2.7182818288

Position Function over Time

Given a position function s (t), the change in position becomes d = rt

Definite Intergral

The Area function/intergral

above represent the Net area

equals TOTAL net area of a function

area below the axis is negation

area aboive the axis is positive

Variable of integration

dx

integrand

function under the integral

Limits of integration / limits of summation

Derivative is the velocity / slope rate of change.

Definition of Area Under a Curve

If the function f is continuous on [a,b] and if f (x) > 0for all x in [a,b], then the area under the curve

Inverse Trig Functions

SOHCAHTOA

Pythagorean Identities

Implicit Differentiation

dy / dx notation

The derivative of y with respects to x

Implicit functions

Equations that are not written in terms of one variable

Some implicit equations cannot be written explicitly

Explicit funtions

Where one variable is defined explicitly in terms of another variable

Derivatives of Trig Functions

Trigonometric Limits: Recall

Quotient Rule

If f and g are differentiable at x and g (x) are not equal to 0, then d / dx f / g = f'g - g'f / g^2

The derivative of the Top times the Bottom minus the derivative of the Bottom times the Top all over the Bottom squared.

Higher Order Derivatives

Assuming y = f (x) can be differentiated as often as necessary, the second derivative of f is f'' (x)

The average velocity is v (t) = change in distance / change in time

This is just the average / slope

s' (t) = v (t)

Derivative of Polynomials and Exponential Function

Sum/Differnece Rule

If f and g are differenetiable at x, then d/dx (f + g)

Constant Multiple Rule

If f is differentiable at x and c is a constant number then d / dx (cf (x)

Power Rule

If n is a any real number, then f (x) = x^n

Constant Rules

If c is a constant number, then f (x) = c