door Amanda Hubbard 12 jaren geleden
460
Math 252 Measurement
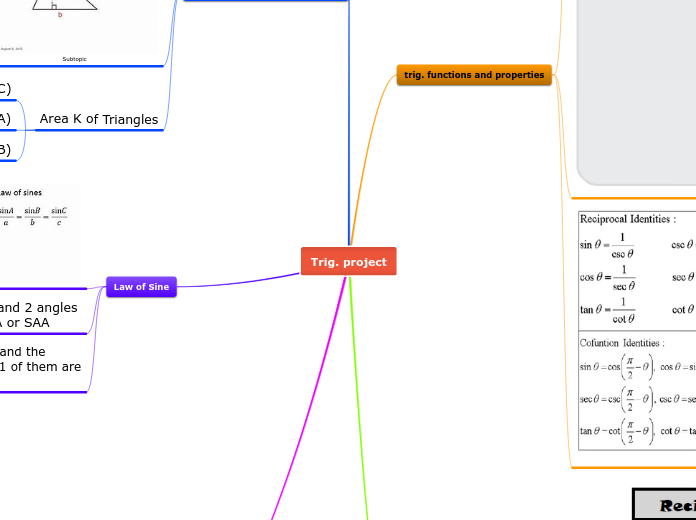
Understanding volume and surface area is crucial when studying the properties of three-dimensional shapes such as prisms, cylinders, cones, and pyramids. For prisms, the volume can be thought of as the base area stacked to the height.