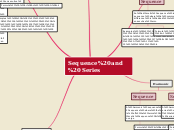
Sequence and Series
Fibonacci
Sequence
The Fibonacci sequence begins
with the numbers 0 and 1.
Each successive number is
the addition of the previous two
numbers in the sequence.
Example :
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, ...
Geometric
A geometric sequence is a sequence where a common ratio is multiplied to each consecutive term.
Formula :
An = A1r^n-1
Examples :
1, 2, 4, 8, 16 r = 2
1, 3, 9, 27, 81 r = 3
1, 5, 25, 125, 625 r = 5
A geometric series is the sum
of all the terms of a geometric
sequence
Formula :
Sn = A1 (1-r^n)
(-------)
( 1-r )
or
Sn = A1 (r^n-1)
(-------)
( r-1 )
Formula : (Infinite)
Sn = A1/r-1
en
Example :
The Cantor Set. What is the length of the Cantor Set? The
easiest way to answer this question is to compute the length of the “middle third” intervals
which are removed at each stage of creating the Cantor Set. The first middle third has length 1/3. The second middle third has length 1/9, but since we remove the middle thirds of two subintervals, the total length removed at the second stage is 2/9. At the third stage we remove four middle thirds, each of length 1/27 , so the total length removed is 4/27 . Since these middle thirds do not overlap, we see that the total length of the intervals removed is:
1/3 + 2/9 +4/27 + 8/81 +.......
Once again, this is a geometric series, with first term a = 1/3 and common ratio r = 2/3. By the formula, the total length L of all these middle thirds is
L= 1/3 + 2/9 + 4/27 + 8/81 + .... = (1/3)/1-(2/3) = (1/3)/(1/3) = 1
If we begin with the unit interval [0, 1] and remove intervals whose total length is 1, the points that remain—in this case, the Cantor set—must have length 1 − 1, or 0. The answer to Question 1 at the end of the Cantor Set Section demonstrates that there are as many
points in the Cantor set as there points in the interval [0, 1]. It boggles the mind to think that they all fit in a space whose total length is zero.
Harmonic
A harmonic series is
a series where the
reciprocals of the terms
form an arithmetic series
and always diverge
Formula:
∞
∑ = 1/n
n =1
Example:
1 + 1/2 + 1/3 + 1/4 + 1/5 + ...
A harmonic sequence is
a sequence where the
reciprocals of the terms
form an arithmetic sequence
and always converge
Formula:
an = 1/n
Example:
1, 1/2, 1/3, 1/4, 1/5
Arithmetic
Series
An arithmetic series is
the sum of all the terms
of an arithmetic sequence.
Formula :
Sn = n/2 (A1+An)
Examples :
1, 2, 3, 4, 5 S5 = 15
16, 33, 50, 67, 84 S5 = 250
1, -3, -7, -11, -15 S5 = -35
Sequence
An arithmetic sequence is
a sequence where a common
difference is added to each
consecutive term.
Formula :
An = A1 + (n-1) d
Examples :
1, 2, 3, 4, 5 d = 1
16, 33, 50, 67, 84 d = 17
1, -3, -7, -11, -15 d = -4
Binomial Series
Statement that for any positive integer n,
the nth power of the sum of two numbers a
and b may be expressed as the sum of n + 1
terms of the form
FORMULA