MAT.116
1.6-1.7
1.7 Rational Exponents and Radicals
Simplifying Radicals
Rationalizing Denominators
When a fraction has a radical in its denominator, we must find an equivalent fraction that does not have a radical in its denominator. This is called rationalizing the denominator.
If the radical is the only term in the denominator, we simply multiply numerator and denominator by the radical in the denominator (or by an appropriate power of the radical).
If the radical has index two and is one of two terms in the denominator, we multiply numerator and denominator of the fraction by the conjugate of the denominator.
Conjugate
Given an expression of the form a+b, we define the conjugate to be the expression a-b.
The product (a+b)(a-b) is a difference of squares and does not include any terms with both a and b in them.
Process
An expression involving radicals is simplified if the following conditions are satisfied:
The powers of all factors under the radical sign are less than the index of the radical.The index of the radical has been reduced as far as possible.No radical appears in a denominator.No fractions appear within a radical.See Table 12 in your text.
Rational Exponents
If n is a natural number and b is a real number, then
b^(1/n) = the nth root of b
If m/n is a rational number reduced to lowest terms (m, n natural numbers), then
b^(m/n) = the nth root of b^m
or
b^(m/n) = (the nth root of b)^m
Properties
The properties of rational exponents are the same as the properties of integral exponents.
Negative Rational Exponents
The rule is the same as for negative integral exponents.
a^(-m/n) = 1/a^(m/n)
nth Roots of Real Numbers
If n is a natural number and a and b are real numbers such that a^n=b, then we say that a is an nth root of b.
When n=2, we typically talk about square roots.
When n=3, we often talk about cube roots.
Notation
Radical
Radical sign
Radicand
Index
Principal nth root
When n is even, we define the principal nth root of a postive real number to be the positive root.
A radical symbol always denotes the principal nth root when n is even.
How many roots?
If n is even, the real nth roots of a positive real number b must come in pairs -- one positive and one negative.If n is even and be is a negative real number, there are no real nth roots of b.If n is odd, then there is only one real nth root of b.See also Table 11 in the text.
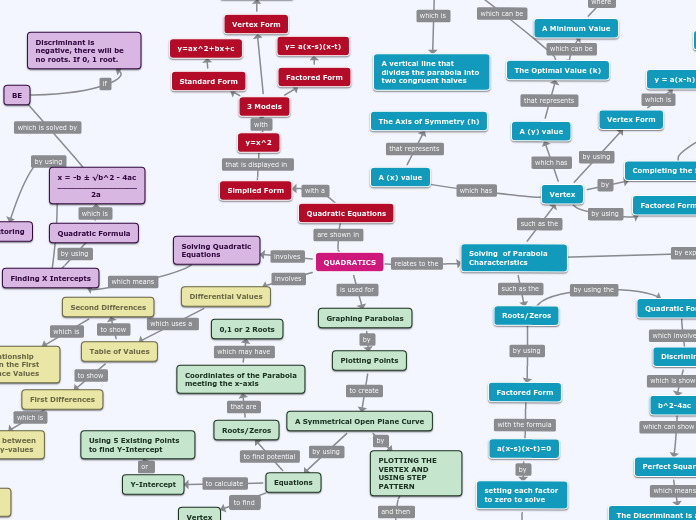
1.6 Solving Equations
Solving for a Specified Variable
Think of all of the variables in the equation as constants except for the variable we are solving for.
Equality Properties of Real Numbers
Let a, b, and c be real numbers.
If a=b, then a+c=b+c and a-c=b-c.If a=b and c is not zero, then ac=ab and a/c=b/c.Let a and be be real numbers and c be a positive integer.
If a=b, then a^c=b^c.Some Special Nonlinear Equations
Rational equations are equations that involve a rational expression. The multiplication property of real numbers is typically used to clear fractions when solving rational equations. However, it is important to check all solutions back into the original equation to make sure that both sides weren't accidentally multplied by zero in that step.
Radical equations are equations that involve a radical expression. The power property of real numbers is typically used to eliminate the radical symbol when solving radical equations. However, it is important to check all solutions back into the original equation to make sure that opposites weren't made equal by an even power in that step.
Linear Equations
A linear equation in the variable x is an equation that can be written in the form ax+b=0, where a and b are constants with a not zero.
A linear equation in x is also called a first-degree equation in x or an equation of degree 1 in x.
Equations
An equation is a statement that two mathematical expressions are equal.
Solving
The standard procedure for solving an equation is to transform the given equation, using an appropriate operation or sequence of operations, into an equivalent equation.
Vocabulary
A variable is a letter that stands for a number belonging to a set of (real) numbers.
A solution of an equation involving one variable is a number that renders the equation a true statement when it is substituted for the variable.
The set of all solutions of an equation is called the solution set.
To solve an equation is synonymous with finding its solution set.