The Six Trigonometric Functions

Mai multe ca aceasta

R-formula and Trigonometry(Sean Group)

după Sean Leow

A Must Know in Trigonometry

după Doris Cruz

Advanced Function

după Wilfred Loh

Unit 1 - Functions: Characteristics and Properties

după Grishma Bk

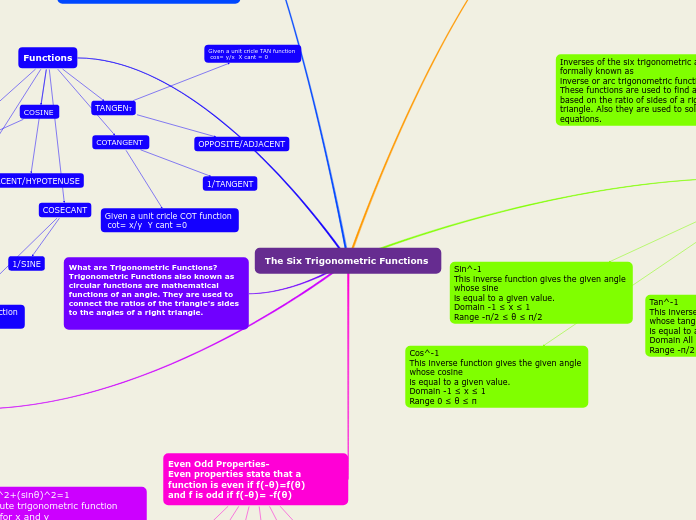
The Six Trigonometric Functions

Inverse Fucntions

Cot^-1 This inverse function gives the given angle whose cotangent is equal to a given value. Domain All real numbers Range 0 < θ < π

Csc^-1 This inverse function gives the given angle whose cosecant is equal to a given value. Domain x ≤ -1 or x ≥ 1 Range -π/2 ≤ θ ≤ π/2 excluding 0

Sec^-1 This inverse function gives the given angle whose sine is equal to a given value. Domain x ≤ -1 or x ≥ 1 Range 0 ≤ θ ≤ π or π < θ ≤ 2π

Tan^-1 This inverse function gives the given angle whose tangent is equal to a given value. Domain All real numbers Range -π/2 < θ < π/2

Cos^-1 This inverse function gives the given angle whose cosine is equal to a given value. Domain -1 ≤ x ≤ 1 Range 0 ≤ θ ≤ π

Sin^-1 This inverse function gives the given angle whose sine is equal to a given value. Domain -1 ≤ x ≤ 1 Range -π/2 ≤ θ ≤ π/2

Inverses of the six trigonometric are formally known as inverse or arc trigonometric functions. These functions are used to find angles based on the ratio of sides of a right triangle. Also they are used to solve equations.

SOHCAHTOA-is the term used to remember the relationship between the trigonometric functions (Tangent,Sine,Cosine) as well as sides of a right triangle.

SOH sinθ= OPPOSITE ______________ HYPOTENUSE

TOA Tanθ= OPPOSITE ________________ ADJACENT

CAH Cosθ= ADJACENT _____________ HYPOTENUSE

Even Odd Properties- Even properties state that a function is even if f(-θ)=f(θ) and f is odd if f(-θ)= -f(θ)

Csc(-θ) ODD

Sec(-θ) EVEN

Cot(-θ) ODD

Tan(-θ) ODD

Cos(-θ) EVEN

Sin(-θ) ODD

Identities- An identity is an equation which for no matter what value you put in for your input there going to be equal.

Pythagorean Identity

(cosθ)^2+(sinθ)^2=1 Substitute trigonometric function values for x and y

tan^2θ+1=sec^2θ

(sin θ)^2+ (cosθ)^2=1 Commutative Property of Addition

1+cot^2θ=csc^2θ

sin^2(θ)+cos^2(θ)=1 Customary notation

Quotient Identity

Reciprocal Identitity

What are Trigonometric Functions? Trigonometric Functions also known as circular functions are mathematical functions of an angle. They are used to connect the ratios of the triangle's sides to the angles of a right triangle.

Functions

COSECANT

1/SINE

Given a unit cricle CSC function csc= 1/y Y can't = 0

SECANT

Given a unit cricle SEC function csc=1/x X cant =0

1/COSINE

SINE

Given a unit cricle SINE function sin = y value

OPPOSITE/HYPOTENUSE

COTANGENT

Given a unit cricle COT function cot= x/y Y cant =0

1/TANGENT

TANGENT

OPPOSITE/ADJACENT

Given a unit cricle TAN function cos= y/x X cant = 0

ADJACENT/HYPOTENUSE

COSINE

Given a unit cricle COSINE function cos= x value

Properties Domain and Range

Secant Properties Range- (-∞, -1] ∪ [1, ∞) Domain- All real numbers except where cos(θ) = 0 Period-2π

Periodic Functions- Any mathematical function that repeats its values over a predetermined period of time or range of values is said to be periodic. The smallest value is called period.

Sine Properties Range- [-1,1] Domain- All real numbers Period-2π

Cosecant Properties Range- (-∞, -1] ∪ [1, ∞) Domain- All real numbers except where sin(θ) = 0 Period-2π

Cosine Properties Range- [-1,1] Domain-All real numbers Period-2π

Tangent Properties Range- (-∞,∞) Domain- All real numbers except where cos(θ) = 0 Period-π

Cotangent Properties Range- (-∞, ∞) Domain- All real numbers except where tan(θ) = 0 Period-π