Math 251- Fractions
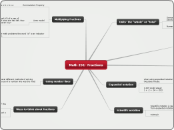
Ways to think about fractions
Iteration- Visualization of repeating equal parts to equal a whole.
Partiioning- This is the visualization of "spllitting up" the whole into whole numbers.
Using number lines
While we learned several different methods of solving fracion problems, the use of a number line was the most helpful for me.
EX: "I had 48 dollars in savings. I spent 2/3 of my savings on new pants. I also spent 1/4 of my savings on iTunes downloads. How much money did I spend? What fraction of my savings did I spend?
First , draw out a number line with 0 at one end and 48 on the other. Then mark the 1/2 mark in the line. If you divide 48 by half you get 24. Half of 1/2 equals 1/4, therefore you divide 24 in half and get 12. Next, divide 48 by 3 to get 1/3 of 48. This equals 16 and 1/3 equals 16. Because you want to find what 2/3 is, you use iteration and multiply 16 by 2. This gives you 2/3 = 32. Then you add 1/4 (12) and 2/3 (32) and get 44. This means you have spent $44. The fraction of savings that you spend is 44/48.
It reminded me of using the ratio table, just on a line.
It keeps me organized and gives me a meaningful visual of partioning.
Multiplying fractions
When looking at math problems the word "of" is an indicator of multiplication.
Area model
The example in class said: Miah had 4/6 of a pan of brownies left. Sarah bought 3/4 of what she had left. How much of the pan of brownies did sarah buy?
So first I drew a picture of a pan with 6 brownies in it. I then crossed out two "brownies" from the pan. The remaining amount of the pan was 4/6. I then shaded in 3/4 of what was left of the 4/6 and it left me with one brownie piece. I then looked at the pan as a whole (including the pieces I had crossed off) and saw that sarah had taken half of the whole pan.
Commutative Property
a x b = b x a
No matter how you multiply you get the same answer! This is useful for kids who find that grouping numbers into easy or friendly parts makes the most sense.
Scientific notation
Subtopic
Scientific notation is really easy after you have converted it from expanded notation.
Expanded notation
1.243 would equal:
1 + .2 + .04 + .003
when using expanded notation it is important to remember the place values.
Main topic
How to say fractions
This strategy is great if you are trying to teach improper fractions. Kids get confused when they see 5/4 because they can't comprehend 7 out of 4. Explaining to kids that they have five 1/4 pieces makes it easier to understand.
Ex: if you have 3/4 you would say
"I have three 1/4 pieces".
Its helpful to say the number of fraction pieces
Units- the "whole" or "total"
Numerator- the number of pieces you have
Demoninator- the total number pieces you could have