作者:Meredith Tillotson 1 年以前
107
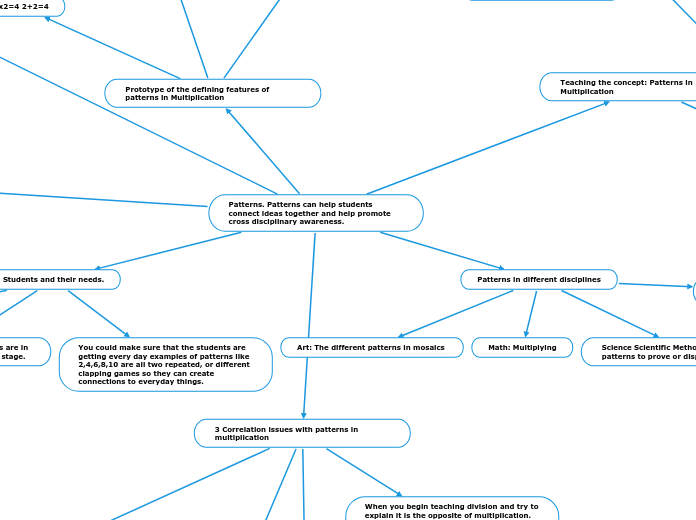
Patterns. Patterns can help students connect ideas together and help promote cross disciplinary awareness.
Students often benefit from recognizing and working with patterns, which can bridge various disciplines and enhance their learning experience. In the concrete operational stage, as per Piaget'