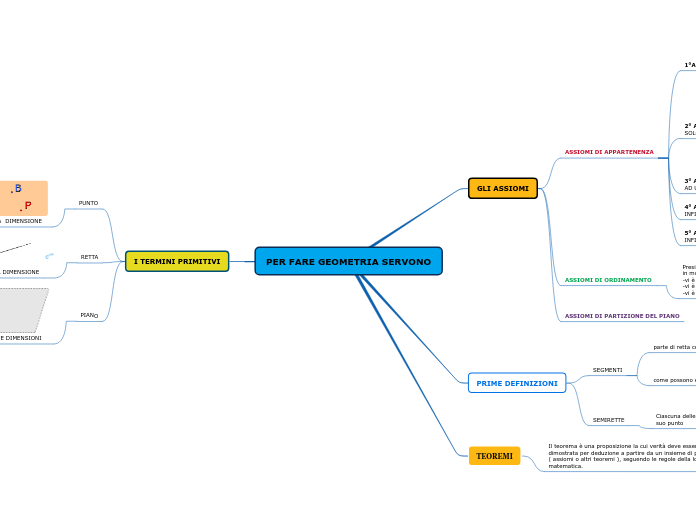
PER FARE GEOMETRIA SERVONO
'Six Thinking Hats' can help you to look at problems from different perspectives, but one at a time, to avoid confusion from too many angles crowding your thinking.
I TERMINI PRIMITIVI
The Blue Hat thinking represents process control. When having trouble because ideas are needed, the Green Hat may come in handy since it is the one used for creativity. In case of emergencies and dealing with them, the Black Hat is required.
PIANO
HA DUE DIMENSIONI
RETTA
HA UNA SOLA DIMENSIONE
PUNTO
NON HA DIMENSIONE
Il teorema è una proposizione la cui verità deve essere dimostrata per deduzione a partire da un insieme di premesse ( assiomi o altri teoremi ), seguendo le regole della logica matematica.
PRIME DEFINIZIONI
SEMIRETTE
Ciascuna delle due parti in cui rimane divisa una retta da un suo punto
SEGMENTI
come possono essere i segmenti?
CONSECUTIVI
ADIACENTI
parte di retta compresa tra due suoi punti
GLI ASSIOMI
Using the white thinking hat, you center your attention around the available data. Take the information that you have, analyze it, and see what you can learn from it. Become aware of your weak points and start working on improving your knowledge.
ASSIOMI DI PARTIZIONE DEL PIANO
ASSIOMI DI ORDINAMENTO
Presi due punti A e B distinti su una retta r orientata disegnati in modo tale che A precede B si ha che: --vi è almeno un punto che precede A
-vi è almeno un punto che segue A e precede B -vi è almeno un punto che segue B
TEOREMI
Per un punto P di un piano passano infinite rette che appartengono al piano
La retta contiene infiniti punti ed illimitata
ASSIOMI DI APPARTENENZA
5° ASSIOMA: LO SPAZIO CONTIENE INFINITI PUNTI INFINETE RETTE E INFINITI PIANI
4° ASSIOMA: OGNI PIANO CONTIENE INFINITI PUNTI E INFINITE RETTE
3° ASSIOMA: SE DUE PUNTI DI UNA RETTA APPARTENGONO AD UN PIANO , L'INTERA RETTA APPARTIENE AL PIANO
2° ASSIOMA: PER TRE PUNTI NON ALLINEATI PASSA UN SOLO PIANO
1°ASSIOMA: PER DUE PUNTI PASSA UNA SOLA RETTA