par MAMADOU BARRY Il y a 9 années
457
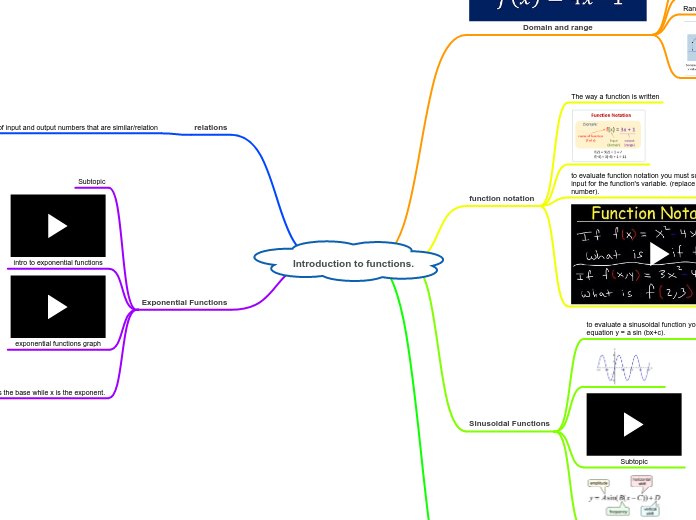
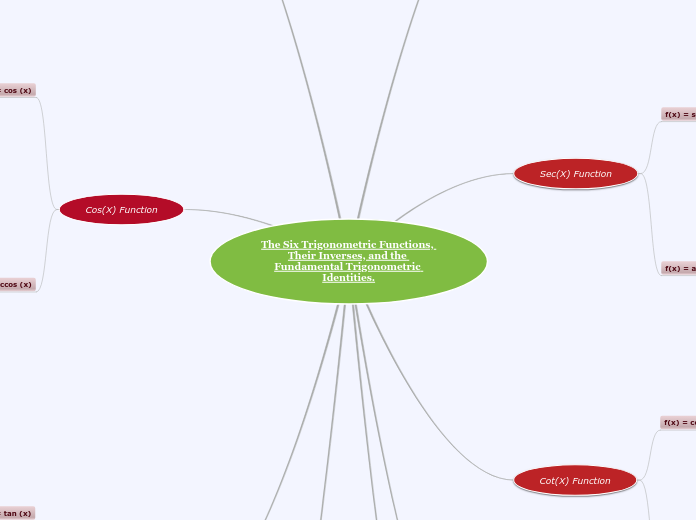
TRIGONOMETRIC FUNCTIONS
The tangent function exhibits specific properties based on its quadrant positioning where it is positive in the first and third quadrants, and negative in the second and fourth quadrants.
par MAMADOU BARRY Il y a 9 années
457
Plus de détails
THE DOMAINE OF THE COSECANT FUNCTION IS THE SET OF ALL REAL NUMBERS EXCEPT INTEGERS MULTIPLES OF π(180 degrees)
• Domain: IR/{X≠Kπ},K is an integer
THE RANGE OF THE COSECANT FUNCTION CONSISTS OF ALL REAL NUMBERS LESS THAN OR EQUAL TO -1 OR GREATER THAN OR EQUAL TO 1. THAT IS
• Range: IR/{Y≤-1 OR Y≥1}
tan(2α) =(2tan2α)/(1-tan^2(α))
tan(α/2) =± √(1-cosα)/(1+cosα)
tan(α+β) = (tanα+tanβ)/(1-tanαtanβ)
tan(α-β) = (tanα-tanβ)/(1+tanαtanβ)
The tangent function is an odd function;
tan(-ϴ) = tan ϴ
by using the pythagorian theorem we can demonstrate that:
tan^2(ϴ) +1 = sec^2(ϴ)
Reciprocal Identity
tan ϴ = sinϴ/cosϴ and cotϴ = cosϴ/sinϴ
hence tanϴ = 1/cotϴ
tanϴ = sinϴ/cosϴ
The domain of the inverse function of tangent is negative infinity to infinity and the range from zero inclusive 90 degree exclusive
the tangent function is an odd function;
tan(-ϴ) = -tan ϴ
The tangent function is:
-positive in the first and third quadrant
-negative in the second and fourth quadrant
The domain of tangent function is the set of all real numbers except odd integers multiple of kΠ
cot(2α) = (1-tan^2(α))/(2tan2α)
cot(α/2) =± 1/√(1-cosα)/(1+cosα)
cot(α+β) = (1-tanαtanβ)/(tanα+tanβ)
cot(α-β) = (1+tanαtanβ)/(tanα-tanβ)
COTANGENT IS AN ODD FUNCTION LIKE TANGENT;
cot(-ϴ) = -cot(ϴ)
using the pythagorian identity
cos^2ϴ +sin^2ϴ = 1 , and by dividing both sides by sin^ϴ, we can obtain:
cot^2ϴ +1 = csc^2ϴ
cotϴ = 1/tanϴ
sin^2ϴ + cos^2ϴ = 1
The periodicity is given by T=2π/w. We know that the graph of y = sin(wx) is obtained from the graph of y = sin(x) by performing a horizontal compression or stretch by a factor of 1/w. This horizontal compression replaces the interval[0,2π], which contains one period of the graph of y = sin(x), by the interval [0,2π/w], which contains one period of the graph of y = sin(wx).
In general, the value of the function
y = A sin(x), where A≠0, will always satisfy the inequality
-|A| ≤ A sin(x) ≤ |A|. The number |A| is called the amplitude of y = A sin(x)
The sine function is an odd function there for ,
sin(-ϴ) = -sinϴ
The sine function is :
-positive in the first and second quadrant;
-negative in the third and fourth quadrant
The domain of the sine function is the set of all real numbers.
The range is the set of all real numbers between -1 and 1 inclusive
sec( α/2) = 1/( √(1-cos α)/2)
sec(2ϴ) =1/(cos^2 ϴ - sin^2 ϴ)
sec(2ϴ) = 1/(1- 2sin^2 ϴ)
sec(2ϴ) = 1/( 2cos^2 ϴ - 1)
FOR THE SECANT AND ITS INVERSE FUNCTION, THE FOLLOWING PROPERTIES HOL:
SEC^-1(SEC X) = X ; [0,pi/2)
SEC(SEC^-1 X) = X ; X LESS THAN or equal to -1 or X greater than or equal to 1
THE SECANT FUNCTION IS NOT A ONE-TO-ONE FUNCTION WHEN WE CONSIDER ITS ENTIRE DOMAIN, HOWEVER, WE CAN RESTRICT THAT DOMAIN TO GET AN INVERSE FUNCTION. If we restrict the domain of
y = sec(x) to the interval [0,π/2), the restricted function y = sec(x) ,
0 ≤ x < π/2 is one-to-one and hence have an inverse function which will be obtained by interchanging x and y in the function
y =f(x) = sec(x) . The implicit form of the inverse is x = sec(y) , 0 ≤ y < π/2 and we obtain
y = sec^-1(x) where
0 ≤ y < π/2
Use sum and difference to establish identities
cos(π/2 -ϴ) = sinϴ
1/cos(π/2 -ϴ) = 1/sinϴ
sec(π/2 -ϴ) = cscϴ
use double angle formula to establish identity
cos^2 ϴ =(1+cos 2ϴ)/2
sec^2 ϴ = 2/(1+cos 2ϴ)
sin^2 ϴ + cos^2 ϴ = 1
divide both side by cos^2 ϴ ,we will have
tan^2 ϴ + 1 = sec^2 ϴ
sec ϴ cos ϴ = 1 hence sec ϴ = 1 / cos ϴ
In general, the value of the function
y = A sec(x), where A≠0, will always satisfy the inequality
-|A| ≤ Acos(x) ≤ |A|. The number |A| is called the amplitude of y = A sec(x)
The periodicity is given by T=2π/w. We know that the graph of y = cos(wx) is obtained from the graph of y = cos(x) by performing a horizontal compression or stretch by a factor of 1/w. This horizontal compression replaces the interval[0,2π], which contains one period of the graph of
y = cos(x), by the interval [0,2π/w], which contains one period of the graph of
y = cos(wx).
Since we want to graph secant in the xy-plane,we shall use the traditional symbol x for the independent variable(or argument) and y for the dependent variable(or value at x).so we write
y=f(x)=sec(x). The independent variable x represents an angle measured in radians.
since the cosine function has period 2π,we only need to graph y=f(x)=sec(x) on the interval [0,2π],the remainder will consist of repetitions of this portion.
THE SECANTE FUNCTION IS AN EVEN FUNCTION WHICH MEANS:
SEC(-ϴ)=SEC(ϴ)
THE SECANTE FUNCTION LIKE THE COSINE FUNCTION IS A PERIODIC FUNCTION WITH PERIOD 2π
SEC(ϴ+2π)=SEC(ϴ)
BETWEEN THE INTERVAL [0,90] DEGREE,
SECANTE OF ANY ANGLE IN THAT INTERVAL IS POSITIVE,
SEC(X)>0
BETWEEN THE INTERVAL [90,180] DEGREE,
SECANTE OF ANY ANGLE IN THAT INTERVAL IS NEGATIVE,
SEC(X)<0
BETWEEN THE INTERVAL [180,270] DEGREE,
SECANTE OF ANY ANGLE IN THAT INTERVAL IS NEGATIVE,
SEC(X)<0
BETWEEN THE INTERVAL [270,0] DEGREE,
SECANTE OF ANY ANGLE IN THAT INTERVAL IS POSITIVE,
SEC(X)>0
THE DOMAINE OF THE SECANT FUNCTION IS THE SET OF ALL REAL NUMBERS EXCEPT ODD INTEGERS MULTIPLES OF π/2(90 degrees)
• Domain: IR/{X≠Kπ/2},K is an odd integer
THE RANGE OF THE SECANT FUNCTION CONSISTS OF ALL REAL NUMBERS LESS THAN OR EQUAL TO -1 OR GREATER THAN OR EQUAL TO 1. THAT IS
• Range: IR/{Y≤-1 OR Y≥1}
Sum to Product formulas
cos α + cos β = 2[cos(α+β)/2 cos(α-β)/2]
cos α - cos β =-2[sin (α+β)/2 sin(α-β)/2]
product to sum formulas
cos α cos β = (1/2)[cos(α-β)+cos(α+β)]
sin α cos β = (1/2)[sin(α+β)+sin(α-β)]
Half angle formulas for cosine
cos α/2 = √(1-cos α)/2
Double angle formulas
cos(2ϴ) = cos^2 ϴ - sin^2 ϴ
cos(2ϴ) = 1- 2sin^2 ϴ
cos(2ϴ) = 2cos^2 ϴ - 1
cos(α-β) = cos α cos β + sin α sin β
cos(α+β) = cos α cos β - sin α sin β
Two functions f and g are said to be identically equal if
f(x) = g(x)
for every value of x for which both functions are defined. Such an equation is referred to as an identity.
Use sum and difference to establish identities
cos(π/2 -ϴ) = sinϴ
use double angle formula to establish identity
cos^2 ϴ =(1+cos 2ϴ)/2
cos(-ϴ) = cos ϴ
sin^2 ϴ + cos^2 ϴ = 1
sec ϴ cos ϴ = 1 hence cos ϴ = 1 / sec ϴ
tan ϴ = sin ϴ/cos ϴ hence
cos ϴ = sin ϴ/tan ϴ
And cot ϴ = cos ϴ / sin ϴ hence
cos ϴ = cot ϴ/ sin ϴ
For the cosine function and its inverse, the followings properties hold:
f^-1(f(x)) = cos^-1(cos(x)) = x ,0 ≤ x ≤ π
f(f^-1(x)) = cos(cos^-1(x))=x, -1 ≤ x ≤ 1
The range of the inverse cosine function is the restricted domain [0,π]
THE COSINE FUNCTION IS NOT A ONE-TO-ONE FUNCTION WHEN WE CONSIDER ITS ENTIRE DOMAIN, HOWEVER, WE CAN RESTRICT THAT DOMAIN TO GET AN INVERSE FUNCTION. If we restrict the domain of y = cos(x) to the interval [0,π], the restricted function y = cos(x)
0 ≤ x ≤ π is one-to-one and hence have an inverse function which will be obtained by interchanging x and y in the function
y =f(x) = cos(x) . The implicit form of the inverse is x = cos(y) , 0 ≤ y ≤ π and we obtain
y = cos^-1(x) where -1 ≤ x ≤ 1 and
0 ≤ y ≤ π
The periodicity is given by T=2π/w. We know that the graph of y = cos(wx) is obtained from the graph of y = cos(x) by performing a horizontal compression or stretch by a factor of 1/w. This horizontal compression replaces the interval[0,2π], which contains one period of the graph of y = cos(x), by the interval [0,2π/w], which contains one period of the graph of y = cos(wx).
In general, the value of the function
y = A cos(x), where A≠0, will always satisfy the inequality
-|A| ≤ Acos(x) ≤ |A|. The number |A| is called the amplitude of y = A cos(x)
Since we want to graph cosine in the xy-plane,we shall use the traditional symbol x for the independent variable(or argument) and y for the dependent variable(or value at x).so we write
y=f(x)=cos(x). The independent variable x represents an angle measured in radians.
since the cosine function has period 2π,we only need to graph y=f(x)=cos(x) on the interval [0,2π],the remainder will consist of repetitions of this portion.
graph see book chapter 6 section 6.4 figure 48 , table 7 page 395
A function f is even if f(-ϴ)=f(ϴ) for all ϴ in the domain of f, function f is odd if f(-ϴ)=-f(ϴ)
for all ϴ in the domain of f.
cos(-ϴ)=cos(ϴ)
proof see book chapter 6 section 6.3 page 389
DEFINITION
A function f is called periodic if there is a positive number p such that, whenever ϴ is in the domain of f, so is ϴ+p
f(ϴ+p)= f(ϴ). ϴ is the argument of the function.
the cosine function is periodic with period 2π
cos(ϴ+2π)=cos(ϴ)
QUADRANT IV
BETWEEN THE INTERVAL [270,0] DEGREE,
COSINE OF ANY ANGLE IN THAT INTERVAL IS POSITIVE,
COS(X)>0
QUADRANT III
BETWEEN THE INTERVAL [180,270] DEGREE,
COSINE OF ANY ANGLE IN THAT INTERVAL IS NEGATIVE,
COS(X)<0
QUADRANT II
BETWEEN THE INTERVAL [90,180] DEGREE,
COSINE OF ANY ANGLE IN THAT INTERVAL IS NEGATIVE,
COS(X)<0
QUADRANT I
BETWEEN THE INTERVAL [0,90] DEGREE,
COSINE OF ANY ANGLE IN THAT INTERVAL IS POSITIVE,
COS(X)>0
THE DOMAINE OF THE COSINE FUNCTION IS THE SET OF ALL REAL NUMBERS, COS(X)
• Domain: IR
THE RANGE OF THE COSINE FUNCTION CONSISTS OF ALL REAL NUMBERS BETWEEN -1AND 1, INCLUSIVE
• Range: [−1,1]
