a Vanessa Felipe 4 éve
263
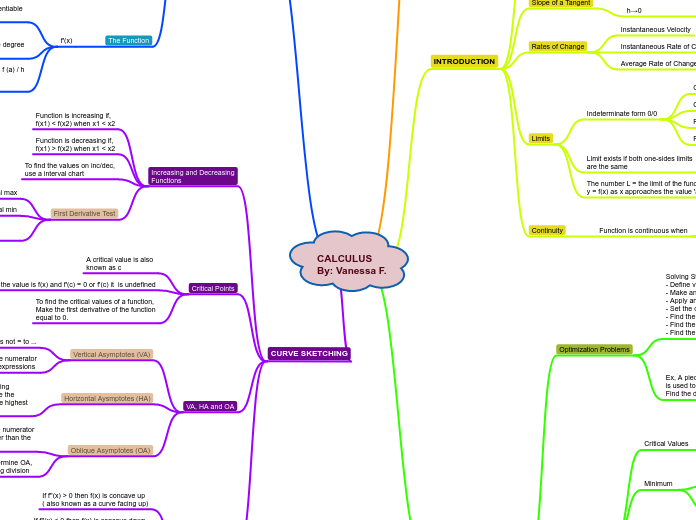
CALCULUS By: Vanessa F.
a Vanessa Felipe 4 éve
263

Még több ilyen
If f'(c) and f''(c) < 0, then local max at c
If f'(c) + 0 and f''(c) > 0, then local min a c
To determine OA, use long division
If the degree on the numerator is one degree higher than the denominator
To determine limits as x approaching infinity of a rational function, divide the numerator and denominator by the highest degree then simplify
Is determined by factoring the numerator and denominator of rational expressions
Domain restriction is, x is not = to ...
If f'(x) sign does not change then the max and min D.N.E at c
If f'(x) changes from - to +, f has a local min
If f'(x) changes from + to - , f has a local max
f'(x) = lim f ( a + h ) - f (a) / h h→0
f'(x) is always one degree less than f(x)
Function f(x) is differentiable only if f'(a) exists
Its not differentiable if f(x) is - Cusp - Vertical Tangent - Discontinuity - Corner
When the slope of a tangent is at 0
f(x) = k, then f'(x) = 0
f(x) =x^n, then f'(x) = nx^n-1
f(x) = k [g(x)], then f'(x) = K [g'(x)]
f(x) = p(x) +- q(x), then f'(x) = p'(x) +- q'(x)
y' = dy/dx
f(x) = f'(x)
f'(x) = n[g(x)] ^n-1 . g'(x)
h'(x)= f'(g(x)) . g'(x)
Lebniz Notation dy/dx = dy/du - du/dx
This is known as the second derivative of the s(t) and first derivative of v(t)
The instantaneous rate of change of the velocity with respect to time
a(t) = v'(t) = s''(t)
-If the object is moving up or right, the v(t) is > 0 -If the object is moving down or left, the v(t) is < 0 - If the object is at rest, v(t) = 0
The instantaneous rate of change of the position function s(t)
v(t) = s'(t)
To find the second derivative, You have to find the first derivative of the function being given.
ex, f(x) = 2x^2 + 5x + 6 f(x) = 4x + 5 f''(x) = 4 Therefore, the second derivative is 4.
Absolute max - the highest point on a graph
Local max - the second highest point on a graph
Absolute min - the highest point on the graph
Local min - the second lowest point on a graph
f'(c) = 0 or f'(c) D.N.E
First I would draw a diagram to better understand the question. Then I would let x represent the width of the removed square. For this particular question, I would use the formula V = (L)(W)(h) I then plug the number given in the question into this formula and find the first derivative. I ten set the derivative to 0. Once i find the critical value, I find my test values and the dimensions.
f(a) is defined
both side limits are equal
limit of f(x) = f(a)
Limit D.N.E if the one-sides limits are not the same
FACTORING if there is a restriction
RATIONALISING if there is a square root
CHANGE OF VARIABLE if there a cube roots or other
ONE SIDED LIMITS if it is a absolute value
< Slope of the secant
< Slope of the tangent
lim f ( a + h ) - f (a) / h
h→0
√a x √a = √a2 = a
(a - b) (a+b) = a2 - b2
( √m - √n ) ( √m + √n ) = √m2 - √n2 = m - n
when there is no denominator, simplify.
MINOMIAL
If the expression is in the denominator, rationalise by multiplying the numerator and denominator by its conjugate.
If the expression is the numerator, rationalise by multiplying the numerator and denominator by its conjugate.
also, tanx = sinx/cosx
ex, y= (sinx + tan x)^4 dy/dx= 4 (sinx + tanx)^3 . (cos + sec^2x) = 4 ( cosx + sec^2 x ) ( sinx + tanx)^3
If g(x) = b^h(x) then g'(x) = b^h(x) . In b . h'(x)
If f(x) = b^x then f'(x). b^x . In b
e^in x= x
The function for this is log power over base