Engineering Physics Option

Még több ilyen

Thermodynamics

Szerző: Siti Nur Hajar Erwan

Newton's Law

Szerző: Emma Penningroth

Equilibrium

Szerző: MacNeil Ella

Equilibrium

Szerző: RYAN MAY

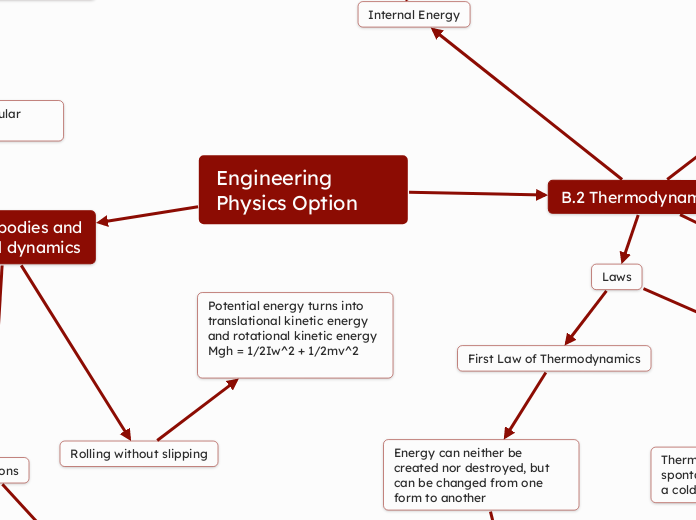
Engineering Physics Option

B.1 Rigid bodies and rotational dynamics

Torque

Torque from couples (opposite direction, same magniute) cause twice the felt torque, meaning it can be multiplied by two.

Clockwise torque opposes counterclockwise rotation Clockwise can be considered positive Counterclockwise can be considered negative

T=Frsin(theta)

r is distance from force applied to the centre of rotation (point)

This can be considered any point, meaning torque will be at equilibrium when considering at any point

Torque is zero if it goes through the axis of rotation

Rotational force, the component that is perpendicular from the line of action through the point

Rolling without slipping

Potential energy turns into translational kinetic energy and rotational kinetic energy Mgh = 1/2Iw^2 + 1/2mv^2

Equations

Conservation of angular momentum

Angular momentum is conserved unless acted upon by an external torque.

The area under a torque-time graph is equal to the change in angular momentum

Rotational and translational equilibrium

Translational equilibrium when resultant force is 0 Rotational equilibrium when the resultant torque on an object is 0

Moment of inertia

Ability to resist changes to its rotational motion

Sum of the mass of all the particles in an object multiplied by their distance to the axis squared

Rotational analog of mass

B.2 Thermodynamics

Internal Energy

Internal energy is the sum of the random kinetic energy of the molecules and their total potential energy. Since there are no IMFs in a gas under the kinetic molecular theory, there is no potential energy. Internal energy is equal to total KE of molecules summed.

Thermodynamic Cycles

A sequence of processes which change the pressure, volume, and temperature of a gas, but eventually returns it to its initial state

The work done by a thermodynamic cycle is equal to the area enclosed by the shape formed by the cycle on a pV graph

Carnot Cycles

Isothermal expansion, Adaiabtic expansion, Isothermal compression, adiabatic compression

No heat engine operating between two reservoirs can be more efficient than a Carnot engine operating between the same reservoirs

The efficiency of a cycle is equal to the ratio between the useful work done and the energy put into the cycle

ncarnot = 1 - Tcold/Thot

Entropy

Entropy increases when heat is added and decreases when heat is removed

Change in entropy is defined as the change in heat of a system divided by its temperature (when temperature is constant)

Change in S = change in Q/T

Measure of the “amount of disorder” present in a thermal system

Types of Processes

Graphical Representation (adiabatic is steeper than isothermal)

Adiabatic

No thermal energy is transferred between the gas and its surroundings (Q = 0) Process must be rapid and the gas must be well-insulated

Isovolumetric

Volume remains constant, no work is done by or on the gas (W = 0)

Isobaric

Pressure remains constant

Isothermal

Temperature (and internal energy) remain constant (ΔU = 0) System must be in contact with a large reservoir (something outside the system that either provides or absorbs heat) and the process must be slow

Sign Conventions

Work Done

W = F * d cos(theta)

In a gas cylinder, W = pressure*A * displacement (x)

W = P * change in V

Laws

Second Law of Thermodynamics

Kelvin

A consequence of this is that the entire universe will eventually be filled with degraded (useless) heat

In a cyclic system, it is impossible to completely convert heat to work

Clausius

Thermal energy cannot be spontaneously transferred from a cold body to a hot body

Planck

Any process which appears to locally decrease the entropy of a system (a fridge makes air cool) is always accompanied by an increase in entropy elsewhere (the fridge’s coolant pump gets hot)

In an irreversible process, the total entropy of an isolated system increases, and in a reversible process, the total entropy of an isolated system remains unchanged

Thermal energy always flows spontaneously from a hot to a cold body

First Law of Thermodynamics

Energy can neither be created nor destroyed, but can be changed from one form to another

If a quantity of energy Q is supplied to a gas, it can either gain internal energy or do work Q=change in U+W