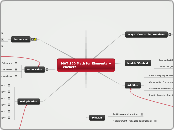
Cartesian Product
Characterized by finding all possible pairings between 2 or more sets of objects.
Continuous
Characterized by repeatedly adding a quantity of continuous quantities. A specified number of times.
MEAUREMENTS
ex. 50mph for 3 hours.
Area Model
Characterized by a product of two numbers representing the sides of a rectangular region such that the product represents the number of unit sized squares within the rectangular region.
Discreet
As repeated addition
There are 3 rows on a bus, there are 4 children in each row. How many children are there in the bus?
Different ways to Add
Left-to-Right
Scratch Method
Low Stress
Any Column First
Speaks for itself.
Lattice Method
Properties of Subtraction?
If a is an element of w then a-0=a=0-a
Counter Example
a-0=a Let a=1 then 1-0=1
but 0-a doesn not equal a because 0-1=-1
Commutaive Property
If a is an element of w and b is an element of w
then a-b=b-a
Counter example
Let a=1 and b=3
a-b=-2 b-a=2
so a-b does not equal b-a
Closure Property
If a is an element of w and b is an element of w,
then (a-b) is an element of w
Counter example
Let a=1 b=3 then 1-3 is not an element of w
MAT 156 Math for Elementary Teachers
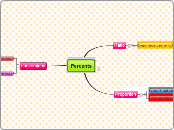
Multiplication
Zero Property
a is an element of w
0 x a= 0
Distributive Property
a is an element of w
b is an element of w
c is an element of w
a(b+c) = ab+ac
Identity Property
a is an element of w
1 x a= a
1 is the identity element
Associative Property
a is an element of w
b is an element of w
c is an element of w
(a x b) x c = a x (b x c)
Commutative Property
a is an element of w
b is an element of w
ab is an element of ba
Closure Property
a is an element of w
b is an element of w
ab is an element of w
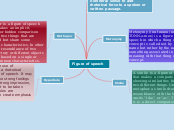
Subtraction
Subtraction is inverse operation of addition.
Four fact families
3+7=10
7+3=10
10-3=7
10-7=3
Missing addend
Characterized by the need to determine what quantity must be added to a specified number to reach some targeted amount.
ex. Tim has 1 ipod. After christmas Tim has 3 ipods. How many ipods did he get for christmas?
Comparison
Characterized by a comparison of the relative sizes of two quantities and determing either how much larger or how much smaller one quantity is compared to the other.
ex. Jonny has 3 baseball cards. Steve has 5 baseball cards. How many more baseball cards does Steve have than Jonny?
Take-away
Characterized by starting with some initial quantity and removing/taking away a specified amount.
ex. Sally had 4 apples, she eats 2 apples. How many apples does sally have now?
Sequences
Sequence: Ordered list of objects, events, or numbers.
Recurrence Relationship Sequences
Current term is dependent on previous term(s)
Geometric Sequences
We can write a rule if we know -The first term (a1) and -The common ratio ( r )
Arithmetic Sequences
We can write a rule if we know -The first term (a1) and -The common difference(d)
Problem Solving
The 4 Steps"How to solve a Problem"
Check your Answer
Implement your Strategy
Devise a Plan
What Strategy are you going to use?
Examples
-Act it out -Draw it out
-Look for a pattern -Guess & check
Understanding the Problem
Division
Measurement (repeated subtraction)
Characterized by using a given quantity to create groups (partitions) of a specified size (amount) and determining the number of groups (partitions) that are formed.
KNOW
-Quantity starting with
-Size of each group
FIND
-Number of groups
*Twenty-five students must be grouped into groups of 5. How many groups of students can be made?
Partition (equal sharing)
Characterized by distributing a given quantity among a specified number of groups (partition) and determing the size (amount) in each group.
KNOW
-The quantity we're starting with
-Number of groups
FIND
-Size of each group
*Twenty-four pieces are to be shared among 6 students. How many pieces of candy will each student get?
Addition
Identity Property of Addition of Whole Numbers (w)
If a is an element of w then a+0=a=0+a The identity element for addition is 0.
Associative Property of Whole Numbers (w)
If a is an element of w, b is an element of w and c is an element of w, then (a+b)+c=a+(b+c)=(a+c)+b
Commutative Property of addition on whole numbers (w)
If a is an element of w and b is an element of w, then a+b=b+a
Closure Property of Additive
If a is an element of x and b is an element of x, the a+b is an element of x.
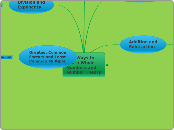
Models/Context
Continuous (Numberline) Model
Measured Quantities
Time, Distance, Area, Volume
Characterized by the combining of two continuous quantities.
Discreet (set) Model
Counted Quantity
Markers, Feet, Chairs, Desks
Characterized by combing two sets of discreet objects.
Discreet- Indidually seperate and distinct objects.
Ways of Recording Numbers
0 is not a counting number.
Hindu-Arabic
Usage of digits/numerals and place value.
Roman System
Babylonions
Mayan
Egyptian
Tally System
Works for smaller amounts