Quadratics Concept Map Assignment

もっと見る

Problem Solving

Ashley Volkenantにより

Algebra Ladder Word Splash

Emily Hodgeにより

MAT.116 Overview

David Kedrowskiにより

Browser

Mike Tonにより

FOIL Method

First Outside Inside Last ( x + 3 ) ( x + 2 ) = x^2 + 2x + 3x + 6 = x^2 + 5x + 6

Distributive Property

a(b + c) = ab + ac e.g. 2(x + 4) = 2x + 8

( x + a ) ( x – a ) = x^2 – a^2

( x + 5 ) ( x – 5 ) = x^2 – 5x + 5x – 25 = x^2 – 25 Middle term cancel out

Sub. in x and a values to expand

x^2-5^2 =x^2-25

( x + a )^2 = x^2 + 2ax + a^2 ( x – a )^2 = x^2 – 2ax + a^2 When the Binomial is square, to expand you must multiply the binomial by itself

2. Use FOIL Method

1. Get rid of the square sign and have the binomials multiply eachother ( x – 3 ) ( x – 3 )

( x + 5 )^2 = ( x + 5 ) ( x + 5 ) = x^2 + 10x + 25

Expanding Binomials

(a + b)(c + d) = ac + ad + bc + bd e.g. (x + 4)(x – 3) = x^2 – 3x + 4x – 12 = x^2 + x – 12

a^2 - b^2 = (a+b)(a-b) 144p^2 - 81 = (12p+9)(12p-9)

(12p+9)(12p-9) is a factored difference of squares

2. Factor by Grouping (if necessary)

Group terms with common factors to solve

2x^2+6y+4x+3xy =2x^2+4x+6y+3xy =2x(x+2)+3y(2+x) =(x+2)(2x+3y)

a = vertical stretch/compression factor

Use Step Pattern for plotting points of parabola

Step Pattern -------------- 1,3,5,7,9,....

Multiply a value and step pattern to get correct points of a given parabola

Ex. a=2 Step Pattern =2[1,3,5,7,9] =2,6,10, 14, 18

Direction of Opening ----------------------- If a>0 it is upward opening parabola, if a<0 it is downward opening parabola

If -1 < a < 0 or 0 < a < 1, (a is a fraction)then the graph is compressed vertically

If a > 1 or a < -1, then the graph is stretched vertically by a factor of a

Vertex (h,k)

Vertex --------------------------------------- (Axis of Symmetry, Optimal Value) (x, y) (2, -8)

Vertex Form

y = a(x-h)^2 + k

Base Parabola is y=x^2

Sub in x=0 to find y-intercept

k = y value of the vertex

"k" value is added

"k" represents a vertical shift

If k>0, it is a vertical shift up by k units. If k<0, it is a vertical shift down by k units.

h = x value of the vertex and axis of symmetry

"h" value is subtracted

"h" represents a horizontal shift

If h>0, it is a horizontal shift to the right. If h<0, it is a horizontal shift to the left

Factored Form

y = a(x-r)(x-s)

Sub in x as 0 to find y-intercept

y=0.5(x-6)(x+2) y=0.5(0-6)(0+2) y=0.5(-6)(2) y=0.5(-12) y=(-6) Y-intercept is (0,-6)

Sub in axis of symmetry as x value to find optimal value (y value of vertex)

y=0.5(x-6)(x+2) y=0.5(2-6)(2+2) y=0.5(-4)(4) y=0.5(-16) y=(-8) Optimal Value of parabola is -8, meaning the y-value of the vertex is -8

Binomials (x-r) and (x-s) gives the x-intercepts

Solve each binomial individually for x to get the x-intercept(s)

y=0.5(x-6)(x+2) -------------------------- x-6=0 x+2=0 x=6 x=-2 x1= (6,0) x2= (-2,0)

adding x-intercepts and then dividing by 2 = axis of symmetry - midpoint of x-intercepts

(6,0) and (-2,0) ------------------ =[6+(-2)]/2 =[4]/2 =2 Axis of Symmetry is (2,0) X value of vertex is 2 - (2,y)=vertex

axis of symmetry is the x value of the vertex

Standard Form

y = ax^2 + bx + c

Formula for Axis of Symmetry -------------------- x = -b/2a y=0.5x^2-2x-6 a=0.5 b= -2 c= -6 x= 2/2(0.5) x= 2/1 x= 2

c value is the y-intercept of parabola

a, b and c are all known values

x is the unknown variable

a value cannot be 0

If FD or SD are not constant, the relation is niether

If Second Differences are constant, there is a quadratic relation

Minimum point if the parabola opens upward (point with lowest y-value)

Maximum point if the parabola opens downward (point with highest y-value)

Axis of symmetry on the x-axis is the x-value of the vertex

Optimal Value is the Y-Value of the vertex

Vertex

The point where the axis of symmetry and the parabola meet at its maximum or minimum value

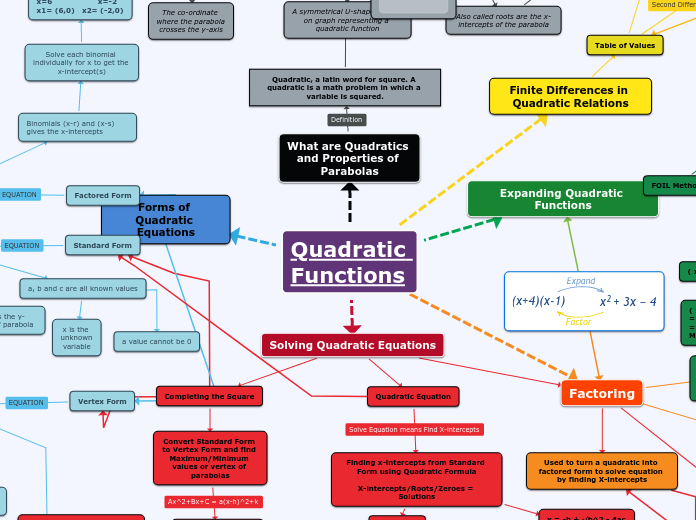
Quadratic Functions

Expanding Quadratic Functions

Solving Quadratic Equations

Quadratic Equation

Finding x-intercepts from Standard Form using Quadratic Formula X-intercepts/Roots/Zeroes = Solutions

(b^2-4ac)

Discriminant

Negative discriminant = no real roots

If discriminant is zero there is 1 root

Positive discriminant = 2 roots

x = -b ± √b^2 - 4ac ________________ 2a

2=-3x^2+4x+2

1. Make sure L.S or R.S is equal to 0

0=-3x^2+4x+2

5. Solve for x2

4. Repeat except make the addition sign between -b and square root subtraction

3. Solve for x1

a=-3, b= 4, c= 2 2. Sub in a, b, and c values in formula

Completing the Square

Convert Standard Form to Vertex Form and find Maximum/Minimum values or vertex of parabolas

y=3x^2-12x-5 y=(3x^2-12x)-5 y=3(x^2-4x)-5 -4/2=-2^2=4 y=3(x^2-4x+4)-5 y=3(x^2-4x+4)-17 y=3(x-2)^2-17

8. Write out the final Equation that is left over

7. Keep the "a" value outside bracket and square root the first term x^2, keep the sign of the middle term (addition or subtraction) and square root the last term in bracket, add a square outside of end bracket to give you: (x-h)^2

5. Move the opposite sign (b/2)^2 value outside bracket by multiplying it by "a" value

6. Add this value with the k value outside bracket to get final k value

4. Add the opposite sign of the (b/2)^2 inside the bracket EX. 2(x^2+6x+9-9)+11

3. Make Perfect Square Trinomial inside bracket using: (b/2)^2

2. Common Factor the "a" value

1. Put brackets around ax^2+bx terms

Vertex = (2,17) Minimum = -17

Factoring

Difference of Squares

a^2 - b^2 = (a+b)(a-b)

a^2 = 144p^2 so a = √144p2 = 12p b^2 = 81 so b = √81 = 9

Sub. in a and b into reference equation to get factored

Ax^2+Bx+C =144p^2+0x-81 =144p^2-81 =(12p+9)(12p-9)

Identifying Characteristics

Contains Perfect Squares

Contains a difference (Subtraction)

Contains Binomials (2-terms)

Perfect Square Trinomials

Same method of factoring is used as factoring trinomials except you write the product as the square of a binomial

First and last terms are perfect squares

a^2±2ab+b^2 = (a±b)^2

Check that "2ab" is the middle term

2ab = 2(2x)(3) = 4x(3) = 12x

Therefore, 2ab is the middle term and that means it is a square trinomial

Using this as a reference equation find out what a and b are

a^2 = 4x^2 so a=√4x^2 = 2x b^2 = 9 so b=√9 = 3

4x^2+12x+9 4(9) = 36 m(n)=36 m = 6 n+n=12 n = 6 Sub. in numbers as bx term = 12x =4x^2+6x+6x+9 =2x(2x+3)+3(2x+3) =(2x+3)(2x+3) =(2x+3)^2

6. The last two binomials should be the same and so you simplify them together by writing the product as the square of the binomial

E.g. (2x – 3)(2x – 3) = (2x – 3)^2

5. Group the terms with common factors and factor each binomial group

4. Substitute the two numbers for the middle term

3. Multiply a value and c value -Find two numbers that multiply to the product of a(c) and have the sum of b

2. Move GCF outside of bracket

1. Factor out the GCF by dividing it with all terms

Finding what to multiply together to get an expression

Trinomial Standard Form ax^2+bx+c ---------------------------- 2x^2+10x-12 =2(x^2+5x-6) =2(x^2-1x+6x-6) =2(x(x-1)+ 6(x-1) =2(x-1)(x+6)

x-1=0 x+6=0 x=1 x=-6

If the trinominal cannot be common factored and the a value is more than 1, you have to multiply a and c

m(n)=a(c) m+n=b

4. Factor by grouping

3. Find Two integers that multiply to "c" and add to "b"

m*n=-6 m+n=-5 -1*6=-6 -1+6=5

Sub. in these numbers for b value

These two integers end up being (x-r) and (x-s) in factored form

1. Common Factor (If Any) Common Factor = 2

Binomial Common Factor

2(x+1)-3y(x+1) _____________ (x+1) = (x+1)(2-3y)

Monomial Common Factor

12 x^2y-9x^3y^2z+18x^2y^2 _________________________ 3x^2y =3x^2y(4-3xyz+6y)

Divide each term by the GCF and write what remains in bracket

Move GCF outside of bracket

Identify greatest common factor = 3x^2y

Used to turn a quadratic into factored form to solve equation by finding X-intercepts

Finite Differences in Quadratic Relations

Table of Values

SD = Subtracting FD values

FD = Subtracting consecutive y-values

If First Differences are constant, there is a linear relation

What are Quadratics and Properties of Parabolas

Quadratic, a latin word for square. A quadratic is a math problem in which a variable is squared.

Parabola

Optimal Value

The lowest or highest value/peak or bottom of the parabola depending on the a value - UPWARD or DOWNWARD?

Y-intercept

The co-ordinate where the parabola crosses the y-axis

Axis of Symmetry

Axis of Symmetry is the sum of the roots divided by two

A vertical line that divides the parabola into two equal halves

Zeroes

Also called roots are the x-intercepts of the parabola

Can have one, two or zero roots

A symmetrical U-shaped curve on graph representing a quadratic function

Forms of Quadratic Equations