mat151

Mais informações

Concept Mapping

por Fabian Hayles

Attitude And Behavior

por Lara El Bachouti

The Five Research Methods

por Diana saleh

Attitude

por Muhammad Hasnan Mohd Salim

Denice Ceniceros Algebra

3.5

Transformation of a graph

1. Great auntie- tries to stay near you, go the other direction (parenthesis)
2. Example: (x-4) Go to the right 4, opposite of what you are told
3. Elevator man- wants to get a tip so follows the same direction as in the equation
4. Example: x+5 Go up 5 like the equation says to
5. 
   

3.3

More with imaginary numbers

Note: i= Sqrt -1 , i^2=-1

If a>0 , then sqrt -a = i sqrt a

1. Simplify

Example: sqrt -16 Example: sqrt -3 X sqrt -3 | isqrt-3 X -isqrt3

=i sqrt 16 sqrt 9 | i^2sqr3 Xisqrt3

=4i =3WRONG | -1 (3) = -3 RIGHT

Example: 2 +_sqrt-24 Example: sqrt -2 X sqrt -8

2+i sqrt 24 /2 =i sqrt 2 X sqrt 8

2+isqrt 24 /2 i^2 sqrt 16

2+i sqrt 4 sqrt -1 (4)

=-4

i = sqrt -1 i^2=-1

• treat like a variable

Example: i+i=2i Example: 5i-3i=2i

Example: 5i X 3i= 15i^2 = 15(-1) =-15

Example: 5(-4i)= 20i Example: -6(2i-1)=12i+6

Example: 3i/21= 3/2 Example: (2i+1) (2i-1)= 4i^2-2i+2i-1

=4i^2 -1=4(-1)-1= -5

Complex Conjugates

1. (2-3i)^2 2. -1(5-2i)^2

Check Notebook

Complex Conjugates

3 . 4+i/5-i X 5+i/5+i = 20+5i+4i+i^2/ 25-i^2

= 20+9i-1/25+1 = 19+9i/26

Complex Numbers and Exponents

i= Sqrt -1 , i^2=-1 , i^3 = i^2 X i =-1 X i =-i

i^4 =1 , i^5=i^4 X i =i , i^6= i^4 X i^2= -1

i^12= (i^4)^3= 1^3=1 i^50=(i^5)^10= i^10

=(i^2)^25=(-1)^25 = -1

1. i^10 2. i^30 3.i^57 3.

3.2

Quadratic Formula

x= -b+- sqrt b^2-4 ac/2a

Graphically- X intercepts

(2 of these)

(1 of these)

Analysis

the Discriminant b^2-4ac

b^2-4ac > 0 2 real solutions

b^2-4ac=0 1 real solution

b^2-4ac <0 2 imaginary solutions

As long as the equation is of the form ax^2+bx+c=0 , I can use the quadratic formula.

Other ways to solve

1. Complete the square when in standard form. (changes to vertex form)

Example 1: x^2 -8x +9 =0 1. Move the constant to the other side

x^2 -8x=-9 2. (b/2)^2 (8/2)^2=16

(x^2-8x +16)-16 =-9 3. Add and Subtract (b/2)^2

(x-4)(x+4)=-9+16

Sqrt (x-4)^2 = Sqrt 7 4. Put in vertex form

x-4 = +_ sqrt 7 5. Take square root

Example 2: x^2 - 8x=7 IN NOTEBOOK

Example 3: 3x^2 -6x +z=0

When a=/ 1 ...

3x^2 - 6x= -2

3( x^2 -2x ) =-2

3( x^2 -2x +1 -1)=-2

3(x^2-2x+1)+3(-1) =2

3(x-1)^2 = -2+3

3(x-1)^2 =1

(x-1)^2 =1/3

x-1=+_ Sqrt 1/3

x= 1+_ Sqrt 1/3

Factoring when factorable

Example: 2x^2 +2x-11=1

2x=^2+2x-12=0

2(x^2+x-6)=0

2(x-2)(x+3)=0 Divide by 2

(x-2)(x+3)=0

x-2=0 x+3=0

x=3 x=-3

3.1

• Characteristics

1. Degree is 2
2. F(x)=$ax^2+bx+c$ (Standard Form)
3. Graph- shape is parabolic (parabola)

If a>0, then upwards parabola

If a<0, then upside down parabola

Ex: Desmos

D:(-infinity, infinity)

{x|-infin. <x<infin.} - all real #'s

R: [0,inin.)

Increasing: (-infin.,0) Decreasing: (0,infin.)

4 . Verex Form $f(x)=a(x+h)^2+k$

(h,k) vertex

Ex: Find the equation given the vertex and a point on the curve.

V= (0,3) (2,1)

Need to find a and the vertex

1=a(2-0)^2+3

$-2=a(2-0)^2$ Answer: f(x)=-1/2(x-0)^2+3

$-2=a(4)$ f(x)= -1/2 x^2+3

$-1/4 =a$

Find the equation given

V=(-2,4) and (3,6) Answer: F(x)= 2/25(x+2)^2

6=a(3+2)^2+4

2=a(5)^2

2/25=a

Find the function form given standard form

Ex: f(x)=2x^2-4x+1

• x-coordinate of the vertex is -b/2a
• y-coordinate F is (-b/2a)

Ex: b=4 a=2 x= --4/2(2)=1

f(1)= 2(1)^2-4(1)+1= -1 V=(1,-1)

Inequalities

1. One variable $x>_-3$

x+3x-2<4 --> 4x-2<4

4x<6 --> x<6/4=3/2 I.N = (-3,infinity)

2 . Two variables

Graph to find the solution set

y<3x+2 Test Point (-2,3) --> 3<3(-2)+2__ FALSE

3 . 2x+3y>_ -4

1. Test point--(0,0) Answer on Desmos

4 . Absolute Value

|2x|=3

2x=3 or 2x=-3

5 . -2 |5x+10|=-4

-2/-2|5x+10| =-4/-2

|5x+10|<_ 2

5x+10=2 or 5x+10=-2

x<_ -8/5 x>_ -12/5

2.1

2.1 Linear Functions

Types of lines

• y=mx+b - slope-intercept form
• Ax+By=C - standard form
• y-y1=m(x-x1) - point slope

Graphing

y=-2x+3

Parallel Lines-

• Have same slope, different Y intercept

Perpendicular-

• slopes are the opposite reciprocals

Find the equation Given...

1. 2 points
2. (2,5) (1,0)

m= 0-5/1-2= -5/-1 =5

y-0= 5(x-1)

y=5x-5

2 . A point and the slope

Ex: (3,8) m=4/3

y-8= 4/3 (x-3)

y= 4/3x +4

3 . Line parallel to given line

• same slope m= -1/2 gos through (0,0)

y-0= -1/2 (x-0)

y=-1/2x

4 . Line perpendicular

• negative reciprocal of given slope

m= 3/4 m2= -4/3 through (0,-3)

y--3= -4/3 (x-0)

y+3= -4/3x

y=-4/3 x-3

Chapter 2

4.4

Real zeros of higher degree polynomials

Example: $f(x)=x^3-61^2+839x+4221$ reps a

Small country's bird population , x days after May 31st.

Find the date(s) when the population was 5000. Find X when when f(x)= 5000 See Notes

Factoring to find zeros f(x)=ax^2+bx+c

F(x)= x^2-3x+2

0=(x-1)(x-2)

x-1=0 x-2=0

x=1 x=2

Zeros with Multiplicity (factored form) Graph

Example: f(x)=(x+2)^3 Example: F(x)= (x-4)^3(x+1)

=(x+2)(x+2) x=2 =4 Multiplicity

x=-1

Complete Factored Form

a) f(x)= 13x^2 -13x-26 b) f(x)=7x^3-2|x^2-7x+2|

GCF = 13 13(x^2-x-2) GCF= 7 (USe grouping method)

=13(x-2)(x+1) 7(x^3-3x^2)-(x-3)

0=13(x-2)(x+1) x=2 x=-1 7 [(x^3-3x^2)-(x-3)]

7[x^2(x-3)-(x-3)]

7(x-3)(x^2-1)

7(x-3)(x-1)(x+1) X=3,1,-1

C) F(x)=2x^3-4x^2-10x+12 (Use Graph)

Factoring Continued

a) f(x)= 2x^3-2x^2-34x-30 with given zero See Notes

If remainder = 0 ... TRUE FACTOR

Rational Zeros (Not all whole # zeros)

Let f(x)=

4.2

Polynomial Functions and Models

1. Turning Point - Point when it goes from increasing to decreasing/decreasing to increasing _Cubic Function_
2. End Behavior - f(x)--> - infin, x --> - infin

Example: See note book

Intercepts (x and y intercepts)

Example: See notebook x- intercepts (y=0)

zeros

Example: see notebook and desmos

• All intercepts
• End behavior
• Increasing/ Decreasing intervals
• Concavity *Concave up / Concave down*

3 . Piece wise Functions

F(x)= {x x>2 Slope=1

{2 x<_2 y-int=0

^ See notebook

{2x x>0

{x+2 x<0

Project - Compound Interest

FV=PV(1+i)^n

F+P(1+r)^t

#1 and 2# YOU TRY IT

F(x)+ax^2+bx+c F(1+r)^2+b(1+r)+c

4.1 Review

1. Transformations of graphs
2. Translations/ Reflections
3. Minimum
4. Maximum
5. End behavior
6. Increasing/ Decreasing Intervals
7. Domain/ Range
8. Even/ odd functions

4.1

Non Linear Functions

Symbolic Form for a polynomial

Example: y=mx+b OR f(x)=mx+b

F(x)= ax^m+bx^m-1+cx^m-2+. . . .

Graphs

Min and Max , Absolute Min , Absolute Max , Local Min , Local Max

• End Behavior - Tails
• Increasing/ Decreasing
• Domain/Range

Example: $2x^3+3x^2+x-1$ See Desmos

F(x)--> -infinity as x-->- infinity

F(x)--> infinity as x--> infinity

Example: $2x^3+5x^2+x-2$ See Desmos

Minimum - (-1.404,-3.557) Maximum - (0.5,0)

Increasing: ( Decreasing:

Domain: Range:

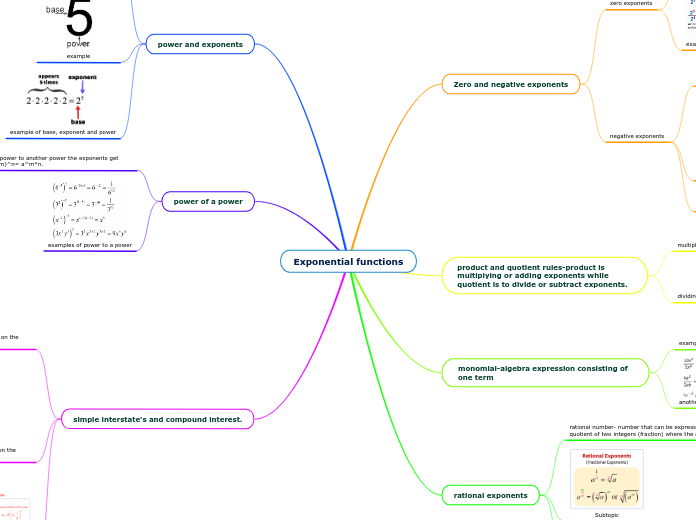
1.4 Types of functions

Types of Functions

Linear

Symbolic Form- y=mx+b (Slope intercept form)Highest exponent degree is 1.Ex: y=3x+1(Vertical Line)x=2 (a line that is NOT function)(Horizontal Line) y=2 (is a function)

Quadratic y=ax^2+bx+cHighest Degree is 2.Ex: $y=x^2+2x+1$1a. $f(x)=3x^2+4x+2$1b. a>02a. a<02b. $f(x)=-x^2+2x+1$

Cubic$f(x)=ax^3+bx^2+cx+d$(a,b,c,d are constants)Highest exponent is 3.$y=x^3$ $f(x)=6x^3$a>0 -- Regular parabolaa<0 -- Upside down parabola

Quartic$f(x)=ax^4+bx^cx^2+dx+e$Degree is 4.Ex:a>0 --a<0 --

Rational$f(x)=a/x-h +k$x-h DOES NOT EQUAL 0Ex:$f(x)=(3/x+2)$

Exponential$f(x)=(a)(b^x)$(a,b are constants)Ex: $a=1$ , $f(x)=3^3$Ex: $f(x)= e^x$( e is a constant)Ex: $a= 1/2$ $f(x)=1/2(2^x)$Logarithmic Absolute Value Functions$f(x)=|x-h|+k$Ex: $f(x)=|x|$Average rate of change- (Slope)$y2-y1/x2-x1=f(x2)-f(x1)/x2-x1$Ex: $f(x)=2x^2$ (1,3) find average{interval} rate of change$f(-2)-f(-3)/-2--3$(-3,-2)Difference Quotient$f(x-h)-f(x)/(x+h)-x$ = $f(x+h)-f(x)/h$ Ex: $f(t)=-16t^2+16t+32$(0,2) $-16t^2+16t+32$f(0)=32f(2)= 0$f(2)-f(0)/2-0 = 0-32/2-0=-32/2=-16$

1.3 Functions

1.3 Functions and their representations

Definitions:

1. Variable- a symbol /letter that represents an unknown number
2. Dependent Variable- "y" output
3. Independent Variable- "x" input
4. Equation- two equal algebraic expressions
5. Relation- comparing at least two things (usually x and y)
6. Function- relation where each input has only one output

1. $y=x^2$
2. () Not equal
3. [] Equal to

Chapter 1