Diff EQ

Mai multe ca aceasta

TV Ch. 27 Change Our Ways 2nd Show 1.6.18

după Nancy Glock-Grueneich

Mimdomo

după Ranj Farhad

Unity3D_Hasten

după Mike Ton

Forms and Function

după grac3311 g

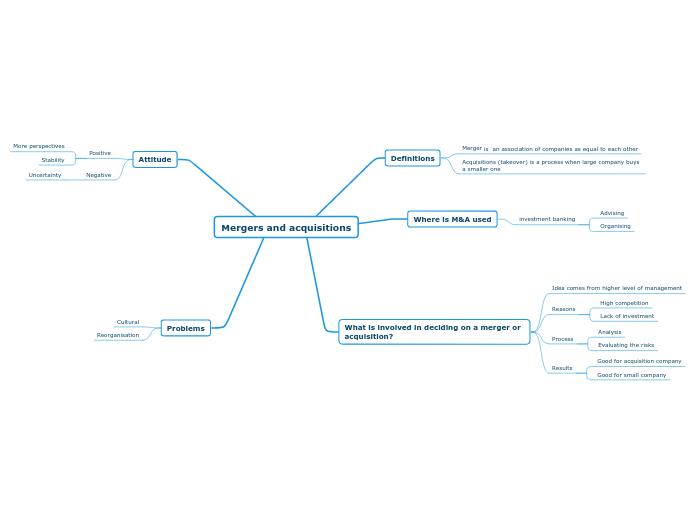
Diff EQ

Systems of diff EQs

eigen values

[a-lamda b c d-lamda]

tTake determinant, solve for lamda, plug in eigenvalue for general solution. If IVP plus in those values and solve for constants

2nd order

Subtopic

particular (LHS)

form based on function: Ae^t, At^2+Bt+C, Acosbt+Asinbt take derivative twice, plug back into RHS to solve for particular

variation of parameters

undetermined coefficients

homogeneous (RHS)

ar^2+br+c=0

repeated: y(t) = c1e^(r1t)+c2te^(r2t)

imaginary: y(t) = e^(at)(c1cosbt+c2sinbt)

real: y(h) = c1e^(r1t)+c2e^(r2t)

Nth order

LaPlace Transform

solve for Y(s), use tables, take inverse etc.

does it exist? (check for domain issues)

is it unique? (check derivative for domain issues)

1st order

integrating factor method

dy/dt + p(t)y = f(t) u = e^∫p(t)dt

Linear nonhomogenous

Find homogeneous and particular solutions

Linear + homogenous?

superposition principle

seperation of variables

(t)dt=(y)dy

directly integrate

isoclines, equilibrium, stability, concavity

application based (model)

competition model

(dR/dt) = R(ar-brR-crS)

tank problem

x'=(r_in)(c_in)-(r_out)(c_out)

Newton's law of cooling

(dT/dt) = k(M-T)

growth/decay

Q = Qo*e^kt