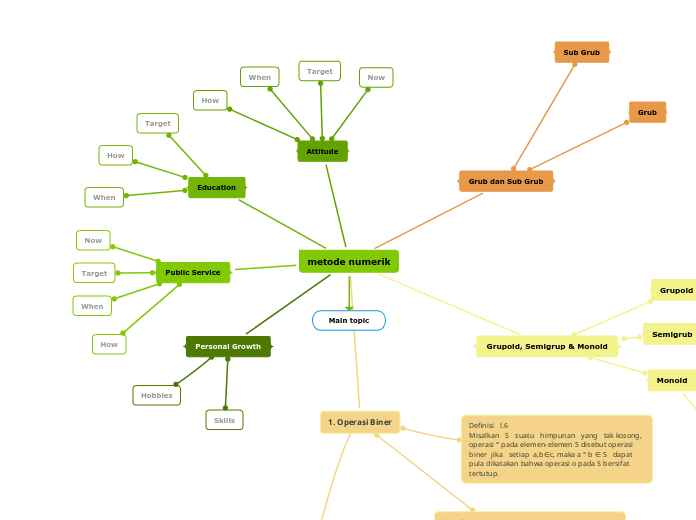
metode numerik
Goal setting is everywhere in our daily lives. We set goals almost every day for our health, career, vacation, etc.
Attitude
We all have annoying traits, we might not be so aware of these but those around us are. Identify these and find ways to remove them your life.
Education
Want to learn something new? It's never too late. Start now!
Public Service
Besides our own wellbeing, we are also a little responsible to leave a good place after us. Think about how you can contribute to your community.
How
When
Target
Now
Personal Growth
This is something that one should work on all their life. We are responsible for our growth. Set your goals! Are there any hobbies that you've always wanted to try? Any skills that you feel you should work on?
Skills
Hobbies
1. Operasi Biner
Everyone has imagined at least once how their life should look like. Try to keep it realistic, plan your future income. After you decide your target, find ways to reach it.
Definisi l.7
Operasi pada S merupakan operasi biner, dapat dikatakan
Komutatif Apabila ∀ a,b ∈ S, berlaku
Asosiatif Apabila ∀ a,b,c ∈ S, berlaku
Memiliki Element Identitas jika ∃ e ∈ S, sedemikian hingga ∀ a∈ S, berlaku
Memiliki Invers jika ∀ a ∈ S,∃ b∈ S, sedemikian hingga dimana b disebut invers dari element a dan ditulis a^(-1)=b
Contoh:
Misalkan Z adalah himpunan semua bilangan bulat. Operasi penjumlahan (+) pada Z merupakan operasi Biner
Penyelesaian:
Z = {…,-3,-2,-1,0,1,2,…}, sehingga sangatlah jelas bahwa operasi penjumlahan (+) pada Z merupakan operasi biner.
Definisi l.6
Misalkan S suatu himpunan yang tak kosong, operasi ° pada elemen-elemen S disebut operasi biner jika setiap a,b∈c, maka a ° b ∈ S dapat pula dikatakan bahwa operasi o pada S bersifat tertutup.
Grupoid, Semigrup & Monoid
Add information on how to improve your overall health. Keep in mind that mental and physical health are both very important! As the saying goes 'Mens sana in corpore sano'!
Monoid
Teorema 2.2.
Misalkan (G,o) adalah suatu semigrup dengan elemen identitas, Apabila suatu elemen G mempunyai invers, maka invers
tersebut tunggal.
Definisi 2.3.
Suatu semigrup yang mempunyai elemen identitas, yaitu ∃ e ∈ G,∀ a∈G berlaku a ° e=e ° a=a maka (G,o) disebut monoid. Suatu monoid yang bersifat komutatif disebut (monoid komutatif) monoid abelian.
Semigrub
Contoh 2.l
Operasi penjumlahan pada Z berlaku ∀ a,b,c∈Z,(a+b)+c=a+(b+c),sehingga (Z,+) adalah suatu semi grup.
Definisi 2.2.
Jika (G,o) suatu grupoid, dan ∀ a ,b ,c∈G berlaku sifat asosiatif, maka (G,o) disebut semigrup.
Grupoid
Teorema 2.l.
Apabila grupoid (G,o) mempunyai elemen identitas, maka elemen identitas itu tunggal.
Definisi
Definisi 2.l. Misalkan G≠∅, dan o adalah operasi biner pada G, maka himpunan G bersama-sama dengan operasi o ditulis (G,o) adalah grupoid.
Main topic
Grub dan Sub Grub
Add information about your career. What position are you in now? Where do you see yourself in the future? Set your goal now! And find ways to climb up the ladder.
Sub Grub
Grub