Quadratics Math Concept map

Meer zoals dit

Quadratic Formula

door Shane Harvey

Quadratic Mind Map

door Patel Sheil

Exponents By: Maryyam-Syed Abdali

door Abdali Maryyam

Quadratics Assignment

door ALI ZEIDAN

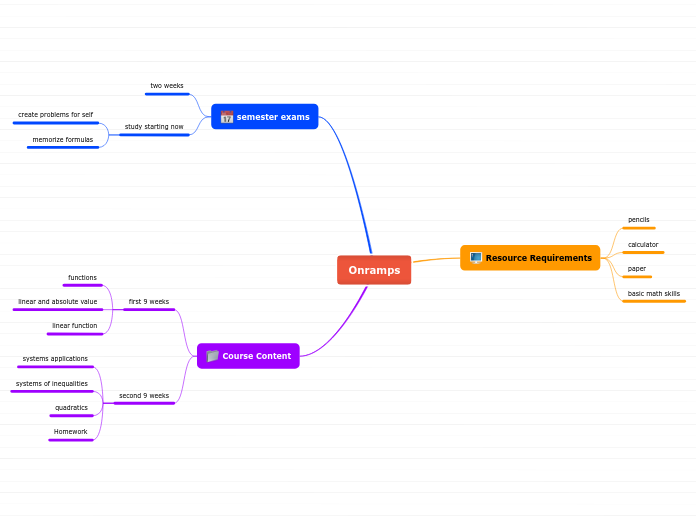
Floating topic

X-intercept /Factored Form

The axis of symmetry is a vertical line that separates the parabola into two congruent halves.

Optimal value

The optimal value represents the y-coordinate

The Axis of symmetry

By using the QUADRATIC FORMULA

Completing the square refers to factoring a perfect square trinomial to get the square of a binomial

Quadratics Math Concept Map

Parabola

y = x²

y = x² points to the coordinates (0,0), which is the origin of the Cartesian plane. The parabola faces upwards.

through a chart using second differences

Second differences, can assists with figuring our whether there is a linear relation, a quadratic relation, or neither.

Key features

Maximum and minimum

Zeroes

Zeros are where a parabola cross the x-axis. A parabola may have one,two, or no zeros

Subtopic

Quadratic Relations

Which includes

X-intercept/Factored Form

y = a(x-p)(x-q)

can also be changed into Standard form

Standard Form

y = ax² + bx + c

complete the square if a ≠1

y = 2x² - 16x -1 y = (2x² - 16x) - 1 y = 2(x² - 8x) - 1 y = 2(x²- 8x + 16 - 16) - 1 y = 2(x² - 8x + 16) - 16(2) - 1 y = 2(x - 4)² - 33

The first blue Line, is the standard form when a ≠1. Group the x-terms as shown in the red line. Common factor just the a-value from the x-terms. Divide the coefficient of the middle term by 2, square it, then add and subtract that number inside the brackets. Remove the subtracted term from the brackets. Multiply it by the "a" value you factored out. Factor the brackets as a perfect square trinomial.

complete the square if a=1

y = x² - 6x +4 y = (x² - 6x) +4 y = (x² - 6x + 9 - 9) +4 y = (x²- 6x + 9) - 9 + 4 y = (x-3)² - 5

The first blue Line is the standard form.The next line shows how the x variables are grouped. After grouping the x variables divide the middle coefficient by two, square the result, than add and subtract the number inside the brackets.Remove the subtracted term, from the brackets.Factor the brackets as a perfect square trinomial. Remember to solve whats outside the brackets as well. This is the result of factoring the standard form, which is now the vertex form.

Vertex Form

y = a(x-h)² + k

a-value

Transformations

The parabola is stretched

More specific on how much the parabola is being compressed or stretched, use the equation, "1a,3a,5a". Substitute the a-values into the equation, as shown below.

The parabola is compressed

The parabola will face upwards

The parabola will face downwards

Translations, which can be either

y = a(x-h)² + k , the k-value is the horizontal translation

y = a(x-h)² + k , the h-value is the vertical translation

The VERTEX(h,k)