по Vita Gamito 3 лет назад
184
Modelling Liner Relations
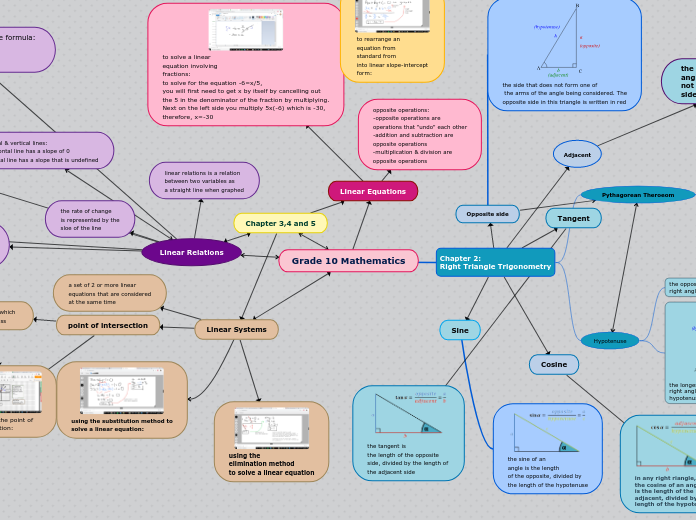
The text focuses on solving linear relations and equations, including the process of isolating variables and rearranging formulas. It outlines methods for handling equations involving fractions and multi-step operations, emphasizing the importance of performing inverse operations in the correct sequence.