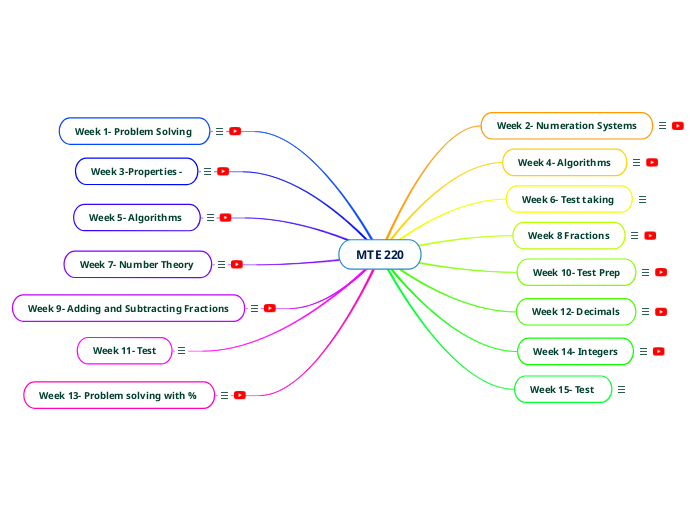
MTE 220
Week 13- Problem solving with %
Is- =
of- x (multiply)
what- n (unknown)
%- decimal
8%- 0.08
Use critical thinking when it comes to problem solving!
a) 8 is what % of 22? (should be around 1/3)
8 = n x 22
22 22
8 divided by 22 equal 0.36 with 36 repeating
Therefore it is 36%
A Students takes a test w/ 45 questions ad gets 37 correct. What % did they get on the test?
- divide 37 by 45
- equals .82 with the 2 repeating so the answer is 82%
7/8 as a decimal
- 7 divided by 8 equals 0.875
Practice problems
In the US, 13 out of 20 cans are recycled. what % of cans are recycled?
- you can either do 13/20 and find the equivalent which is 65/100. 65% is our answer
https://www.youtube.com/watch?v=suAikQqJD34
Week 11- Test
Day 1
Took our test
Day 2
Reviewed tests answers
Week 9- Adding and Subtracting Fractions
Adding fractions with common denominator
examples using pictures will be in video
1/4 + 2/4= 3/4
when both of the fractions have the common denominator, you just need to add the number on the numerators
1/4+1/6=5/12
- because the number in the denominators are different, you have to find the least common nuliptle of the 2 numbers
- 4 and 6 have a LCM of 12
3/12+ 2/12=5/12
If there is a improper fraction, make sure to turn it into a mixed number
12/10-> 1 1/5
Multiplying Fractions
- multiplying fractions decreases the outcome
1/2 of 1/2= 1/4
multiply the numerators together and then multiply the denominators together
Division
2/3 divided by 4/5
do the inverse of the second fraction and then multiply them
2/3 x 5/4= 10/12
https://www.youtube.com/watch?v=ysKCXALX2sM
Week 7- Number Theory
Number Theories
- Types of numbers
- divisibility rules
- Factors
Fractions
a is divisible by b if there is a number c that meets the requirement bxc=a
ex- 10 is divisible by 5 because 2x5=10
Divisibility Rules:
Endings
-by 2: 0,2,4,6,8
-by 5:0,5
-by 10:0
Sum of digits
-by 3: sum of digits is divisible by 3 ex 39- becuase 3+9=12 which is divisible by 3
-by 9: sum of digits is divisible by 9
Last digits
-by 4: last 2 digits are divisible by 4 ex 316
-by 8: last 3 digits are divisible by 8
6
-by 6: if it is divisible by BOTH 2 and 3
7
-by 7: double the last number and subtract the sum by the other numbers ex 826- 6 doubled is 12, then subtract 12 by 82
11
-by 11: "chop off"
Prime factorization
the figerprint or DNA if every composite number:
ALWAYS THE SAME
24 24
6 4 8 3
2 3 2 2 4 2
2 2
GCF; Greatest Common Factor
- List Method
24: 1,2,3,8,12,24,4,6
36:1,2,3,4,6,9,12,18,36
GCF(24,36) : 12
- Prime Factorization Method
24: 2x2x2x3
36: 2x2x3x3
2z2x3 = 12
Least Common Multiple
24:24,48,72,96
36: 36,72
The least common multiple is 72
https://www.youtube.com/watch?v=Df9h5t64NlQ
Week 5- Algorithms
Addition and Subtraction Algorithms
6 Addition algoritms
1.American Standard
256
+415
671
2.Partial Sum- adding from right to leaft and carr
346
+124
10
6
+ 4
470
3.Partial Sum w/ Place Value
346
+124
10
60
+400
470
4.Left to Right- work from the hundreds value to the ones
178
+269
300
130
+17
447
5.Expanded Notation
576 500+70+6
+ 279 +200+70+9
855 855
6.Lattice
5 7 6
+2 7 9
0 1 1
7 4 5
8 5 5
6 Subtraction algorithms
1.American Standard
645
-279
366
2.European/Mexican- instead of taking away from the top place values, you are adding to the bottom place values
3.Reverse Indian
4.Left to Right- solve going from left to right in all place values
5.Expanded Notation-same concept as addition just using multiplication
6.Integer Subtraction- following from right to left and allowing their to be negative numbers. Then solve
https://www.youtube.com/watch?v=TClOCPBYw10
Week 3-Properties -
Properties of addition, subtraction, and multiplication
Addition- to put together/join
Identity Property- a+0=a
ex- 4+0=4 , 3/5+0= 3/5
Commutative (order) Property- the order of the number doesn't matter when adding a+b=b+a
ex- 9+8=8+9
Associative (grouping) Property- grouping in different ways (a+b)+c= a+(b+c)
ex- (3+4)+8 = 3+(4+8)
Subtraction-
- Take away
- ex- 5-3
- Comparison: its not adding or subracting
- ex- how many more coins are in group A than in group B
- Missing addend
- ex- 3+ = 7
Multiplication- repeated addition
3x2 = 3 groups of 2 (2+2+2)
Identity Property of Multiplication- ax1 =a
ex- -3x1= -3
Zero Property of Multiplication- ax0=0
ex- 3x0=0 , 236x0=0
Commutative Property of Multiplication- axb=bxa
ex 3x7=7x3
Associative Property of Multiplication- (axb)xc = ax(bxc)
ex- (2x4)x4 = 2x(4x4)
Distributive Property of Multiplication- multiplying a number by a sum is the same as multiplying each number of the sum then adding them together
ex- 3x(6+1)
(3x6)+ (3x1)
18+3
21
https://www.youtube.com/watch?v=s9h-n95AXrc
Week 1- Problem Solving
Problem Solving
Gorge Playa Theory
- Understand the Problem
- what is it asking?
- do I know what I am looking for?
- Plan how to solve
- trial and error
- act out
- work backwards
- make organized list
- make table
- Implement the plan
- -easier way?
- be patient and persistant
- try different plans
- try different plan
- look back- Reflect
- does this make sense?
- what did you learn?
- was there and easier way to solve?
There are 12 basketball teams in a league. If each of the teams plays each of the other teams once and only play once, how many games take place?
team games
1 0
2 1
3 3
4 6
5 10
6 15
7 21
8 28
9 36
10 45
11 55
12 66
1 cant play itself which is why it has 0 games. On the second row, 1 and 2 play each other for one game. Third row, 1 and 2 played, 1 and 3 played but also 2 and 3 played which is why there are 3 games played. Fourth row we are adding 3 more games because 1 also played 4, 2 played 4 and 3 played 4. this continues with all 12 teams.
We can also see a pattern of adding up another number as each row goes down.
0+1=1
1+2=3
3+3=6
6+4=10
10+5=15
15+6=21
continued
ex- I have four 3-cent stamps and three 7-cent stamps. Using one or more of these stamps, how many different amounts of postage can I make?
Stamps alone (single) 7
stamps together 12 = 19 total
3,3,3,3
7,7,7
37,377,3777
337,3377,33777
3337,333777,3337777
33337,333377,3333777 = 19 total outcomes
https://www.youtube.com/watch?v=zhL3EMFSm6o
Week 15- Test
Test and mindmap
YAAAYUYYYYYY
Week 14- Integers
Positive and Negative Numbers- "the chip method"
- addition
- bring more chips into the circle
- when you are adding a negative number, that creates a zero pair which cancels out
- subtraction
- take ''chips" out of the groups
- which you are subtracting a positive by a negative, you take out the negative out of one of the zero pair +5- (-1) = 6
- multiplying
- +3 x +2 = 6
- 3 groups of 2
- -3 x +2 = -6
- commutative property applies
- when multiplying 2 negatives, "the opposite of" cover the negative of the first integer then use the opposite
- division
- video
- https://www.youtube.com/watch?v=_B1fuzmonO4
Week 12- Decimals
Decimals
1- Thousands
9- Hundreds
4- Tens
3- Ones
.- decimal
5- Tenths
1- Hundredths
3- Thousandths
Smallest-> Greatest
0.3, 0378, 0.98, 0.23
0.23< 0.3< 0.378< 0.98
Adding and Subtracting Decimals
- The decimals have to be lined up on the correct place values
376.25
22.3 This is WRONG
376.25
22.30 This is CORRECT
Multiply
- These numbers do not need to line up
Division
https://www.youtube.com/watch?v=Val4TmjHXRY
Week 10- Test Prep
Test 2 Study Guide
- divisibility rules
- prime numbers- prime factorization tree
- true-false statements
- find GCF and LCM
- fractions- problem solving
Jim, Ken, Len and Max have a bag of bag of mini candy bars from trick or treating together. Jim took 1/4 of all bars, Ken and Len took 1/3 of all the bars. Max got the remaining 4 bars. How many bars were on the bag originally? How many bars did each get?
111 1111 1111 1
3/12 for Jim-12
4/12 for Ken-16
4/12 for Len- 16
1/12 for max- 4 bars
the numbers are over 12 because the LCM of 4 and 3 are 12
https://www.youtube.com/watch?v=kMPhdAXlM8k
Week 8 Fractions
Fractions
1. part-whole
boys/whole class
2.3/4- quotient
3.Ratio
boys/girls
Models
- Surface Area
- 3 out of 4 pieces of pizza are gone
2.Length
3.Set (groups of things)
1 Whole = 4/4, 6/6
-fractional parts are equivalent parts
-the more my whole is divided by. the smaller my pieces get
https://www.youtube.com/watch?v=HBN568uvxi4
Week 6- Test taking
Day 1
The class took the 20 question test for the full class period
Day 2
With our tests being grades- we review frequent questions that were missed by the class
Week 4- Algorithms
Division and Multiplication Algorithms
Division- repeated subtraction
6➗2 division sign
6/2 division or fraction bar
division bar as well
Place Value Explicit
- filling in all of the place values when solving using long division
ex- John has 15 cookies. He puts 3 cookies in each bag. How many bags can he fill
Alternative Algorithm-repeated subtraction
197 cookies and 16 in each box. How many boxes are needed?
197-160- 10 boxes
37cookies left
37-32 2 boxes
5 cookies left
12 boxes in total
Standard Algoritm
Multiplication
Standard Algorithm- multiplying using partial products then adding together
Place Value
23
x14
4x3=12
4x20=80
10x3=30
+10x20=200
322
Expanded Notation
23 20+3
x14 x10+4.
300+ 20+2
322
Lattice- Video explaining
https://www.youtube.com/watch?v=W7cTvEcyyj4
Week 2- Numeration Systems
Numeration Systems
- created to record quantity
- base-10 system
- value changes in position
ex- 348
3- hundreds place
4- tens place
8- ones place
These are place values in base 10
348.27
2- one tenths
7 one hundredths
Base 10
0,1,2,3,4,5,6,7,8,9,10,11,12...
ones
tens
hundreds
Expanded Notation
ex- 348
3 hundreds+ 4 tens+ 8 ones
300+40+8 =348
(3x100)+(4x10)+(8x1)= 348
(3x102 )+ (4x101)+(8x100) =348
Base 5
0,1,2,3,4,105 ,115, 125, 135, 145, 205,...
ones-50
Fives- 51
twenty fives-52
125s- 53
how do you find 2115 in base 10?
(2x52)+(1x51)+(1x50)
(2x25)+(1x5)+(1x1)
50+25+1
56
Base 3
0,1,2,103, 113,123, 203, 213
how do you find 33 in base 3?
27= 1
9= 0
3= 2
1= 0
https://www.youtube.com/watch?v=HJH0gBYhFiA