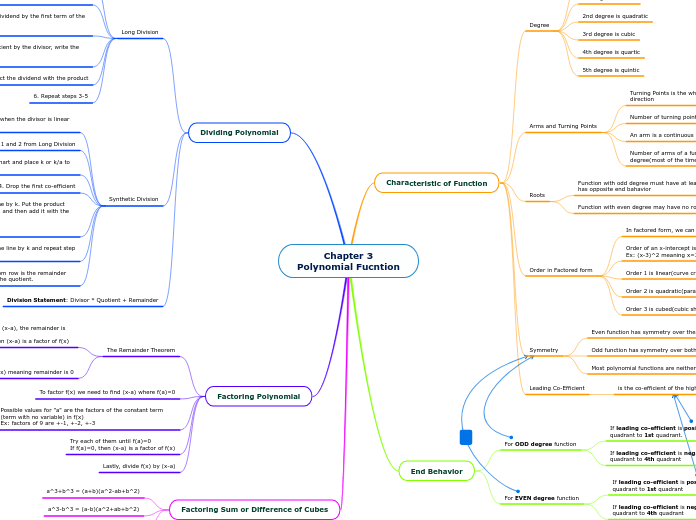
Quadratic Expressions
Expanding
Difference of squares
Follow FOIL method
and remember to cancel
out reciprocals.
(a-b)(a+b)
=A^2+ab-ab+B^2
=A^2+B^2
Squaring binomials
Expand, then follow foil method.
(a+b)^2
=(a+b)(a+b)
=a^2+2ab+b^2
Multiplying Binomials
Follow FOIL method.
(2x+4)(3x+2)
=6x^2+12x+6
Distributive property
Multiply everything inside the
brackets by the number(s) outside of it.
3(2x+4)
=6x+12
Factoring
Difference of Squares
square root both
terms and have a
square outside the
bracket to show it
was factored, expand
both terms, and follow
binomial common factor.
36y^2-100
=(6y)^2-(10)^2
=(6y)(6y)-(10)(10)
=(6y-10)(6y+10)
Perfect Square Trinomials
A^2-2AB+B^2
=(A-B)(A-B)
A^2+2AB+B^2
=(A+B)(A+B)
A=/=1
ax+bx+c
_ * _=ac
_+_=b
A=1
(1)x^2+bx+c
=(x+1)(x+c)
Group Common Factors
Group both sides so to
factor them separately
X^4-2x^3 + 4x-8
Binomial Common Factors
x-3 is multiplied by both 2 and 3y,
making it able to factor out.
2(x-3)+3y(x-3)
=(x-3)(2+3y)
Monomial Common Factors
Both x and y are being
multiplied by 3, making it
able to be factored out.
3y+3x
=3(x+y)